Quadrant Summary Part 12

THE 12 IS FOUR TIMES THREE
https://en.wikipedia.org/wiki/Muffin_tin
There are several standard numbers of cups per tin, including 4, 6, and 12 cups per

A common muffin/cupcake tin

THE MOST COMMON IS FOUR TIMES THREE

https://en.wikipedia.org/wiki/Four_Pests_Campaign

The Great Sparrow Campaign (Chinese: 打麻雀运动; pinyin: Dǎ MáquèYùndòng), also known as the Kill a Sparrow Campaign (Chinese: 消灭麻雀运动; pinyin: Xiāomiè Máquè Yùndòng) and, officially, as the Four Pests Campaign, was one of the first actions taken in the Great Leap Forward in China from 1958 to 1962. The four pests to be eliminated were rats, flies, mosquitoes, and sparrows. The extermination of sparrows resulted in severe ecological imbalance, prompting Mao to end the campaign against sparrows and redirect the focus to bed bugs.

https://en.wikipedia.org/wiki/Four_Olds

The Four Olds or the Four Old Things (simplified Chinese: 四旧; traditional Chinese: 四舊; pinyin: sì jiù) were Old Customs, Old Culture, Old Habits, and Old Ideas. One of the stated goals of the Cultural Revolution in the People's Republic of China was to bring an end to the Four Olds.[1] The campaign to destroy the Four Olds began in Beijing on August 19, 1966, shortly after the launch of the Cultural Revolution.[2]

A single cup within a regular muffin tin is 3 1⁄2 ounces[citation needed] and most often has room for 12 muffins

https://chineseposters.net/themes/four-pests.php

ZECHARIAHS PROPHECY FOUR PARTS

http://www.biblestudytools.com/commentaries/jamieson-fausset-brown/zechariah/zechariah-introduction.html

The prophecy consists of four parts: (1) Introductory, Zechariah 1:1-6 (2) Symbolical, Zechariah 1:7 nine visions; all these were vouchsafed in one night, and are of a symbolical character. (3) Didactic, the seventh and eighth chapters containing an answer to a query of the Beth-elites concerning a certain feast. And (4) Prophetic, the ninth chapter to the end.

https://www.youtube.com/watch?v=2HmfcyWEDY8

CRIS CROSS BRAIDS

CRISS CROSS DUTCH BRAIDS

https://www.youtube.com/watch?v=qyVJdKDt18I

KRISS KROSS TOTALLY KROSSED OUT ALBUM- CROSS/QUADRANT ON THE COVER

https://www.youtube.com/watch?v=Olf1iDWa8rs

THE TETRAHEDRAL KITE WAS INVENTED BY THE FAMOUS ALEXANDER GRAHM BELL- THE NORMAL KITE IS CALLED THE "CROSS KITE" BECAUSE IT LOOKS LIKE A CROSS/QUADRANT

Hargrave's work inspired Alexander Graham Bell to begin his own experiments with a series of tetrahedral kite designs.

https://en.wikipedia.org/wiki/Lawrence_Hargrave

https://en.wikipedia.org/wiki/Tetrahedral_kite

A tetrahedral kite is a multicelled rigid box kite composed of tetrahedrally shaped cells to create a kind of tetrahedral truss. The cells are usually arranged in such a way that the entire kite is also a regular tetrahedron. The kite can be described as a compound dihedral kite as well.

An early design of the tetrahedron kite from Alexander Graham Bell

This kite was invented by Alexander Graham Bell. It came about from his experiments with Hargrave's Box Kites and his attempts to build a kite that was scalable and big enough to carry both a man and a motor. As such, it was an early experiment on the road to manned flight. He worked on the kites between 1895 and 1910.[1] Bell wrote about his discovery of this concept in the June 1903 issue of National Geographic magazine; the article was titled "Tetrahedral Principle in Kite Structure".[2]

From an initial one cell model, Bell advanced to a 3,393 cell "Cygnet" model in 1907. This 40-foot (12.2 m) long, 200 pound (91 kilogram) kite was towed by a steamer offshore near Baddeck, Nova Scotia on 6 December 1907 and carried a man 168 feet (51.2 metres) above the water.

Bell also experimented with a large circular "tetrahedral truss" design during the same period.[3]

The tetrahedral kite, while not easy to make compared to the simple cross kite, is very stable and easy to fly. It flies well in moderate to heavy winds if it is properly set up.

DRAGONBALL Z FOUR STORY ARCS

https://en.wikipedia.org/wiki/Story_arc

The anime Dragon Ball Z adapts four different story arcs from the Dragon Ball manga, each with its own ultimate antagonist, along with original story arcs created for the TV series.

THE FOUR PROTAGONISTS OF THE DESPERATE HOUSEWIVES

https://en.wikipedia.org/wiki/Character_arc

https://en.wikipedia.org/wiki/Desperate_Housewives

The first season premiered on October 3, 2004, and introduces the four central characters of the show: Susan Mayer, Lynette Scavo, Bree Van de Kamp and Gabrielle Solis, as well as their families and neighbors on Wisteria Lane.

The TV series Desperate Housewives made heavy use of character arcs throughout its run, with story arcs (or mysteries, as the show was famed for) normally being used to move the plot along in the background, as the four protagonists, Susan Mayer, Lynette Scavo, Bree Van de Kamp, and Gabrielle Solis, dealt with their various foibles and flaws, through the eyes of their dead friend and neighbour, Mary Alice Young

THE ELITE FOUR IN POKEMON

https://bulbapedia.bulbagarden.net/wiki/Elite_Four

The Elite Four (Japanese: 四天王 The Big Four, lit. Four Heavenly Kings) are four Pokémon Trainers who are regarded as the toughest in their regional Pokémon League, short of the Pokémon League Champion. Those who challenge the Elite Four must have won all eight Badges from that region and face all four and the current Champion consecutively without losing to any of them.

One group resides at the Indigo Plateau in Kanto and are considered the best Trainers in both Kanto and Johto, another is located in Ever Grande City in Hoenn, another is located on Mount Lanakila in Alola and three more reside at Pokémon Leagues in Sinnoh, Unova, and Kalos.

In the games, they employ the use of strong healing items in battle.

The Sinnoh Elite Four in the Pokemon anime

THIS GUN HAS FOUR CHAMBERS

https://en.wikipedia.org/wiki/COP_.357_Derringer

The COP .357 is quite robust in design and construction. It is made of solid stainless steel components. Cartridges are loaded into the four separate chambers by sliding a latch that "pops-up" the barrel for loading purposes, similar to top-break shotguns. Each of the four chambers has its own dedicated firing pin. It uses an internal hammer, which is activated by depressing the trigger to hit a ratcheting/rotating striker that in turn strikes one firing pin at a time. Older "pepperboxes" also used multiple barrels, but the barrels were the part that rotated. The COP .357 operates similarly to the Sharps rimfire pepperbox of the 1850s, in that it uses the ratcheting/rotating striker, which is completely internal, to fire each chamber in sequence.[2]

‘The Card Players’ by Paul Cezanne

16 IN THE CLIP AND ONE IN THE HOLE- 16 SQUARES IN QUADRANT MODEL

https://www.youtube.com/watch?v=60k9Fxrw0us

IT IS A THREE PLUS ONE PATTERN- THERE IS A FOURTH FIGURE IN THE SHADOWS IN THE BACKGROUND- LE NAIN CARD PLAYERS

https://ocherart.wordpress.com/category/banksy/

Quadrant

FOURTH TRANSCENDENT UNSEEN

https://en.wikipedia.org/wiki/File:Syariah-thariqah-hakikah2.jpg

https://en.wikipedia.org/wiki/Sufism

"Tariqat" in the Four Spiritual Stations: The Four Stations, sharia, tariqa, haqiqa. The fourth station, marifa, which is considered "unseen", is actually the center of the haqiqa region. It is the essence of all four stations.

IBN ARABIS WORKS ARE FULL OF QUATERNITIES. HE ORGANIZED HIS WORKS INTO FOURTEEN CHAPTERS BECAUSE HE SAW FOURTEEN AS THE TETRACTYS PLUS FOUR, AND THUS THE MOST PERFECT NUMBER. GREGORY THE GREAT ORGANIZED HIS WORKS INTO FOURTEEN CHAPTERS FOR THE SAME EXACT REASON

Despite time being imaginary, Ibn ‘Arabi considers it as one of the four main constituents of nature: time, space, the monad ( al-jawhar ), and the form {al- ‘arad). Like some modern theories, Ibn ‘Arabi also considers time to be cyclic, relative and inhomogeneous.

FOUR MOTHERS OF EXISTENCE

https://archive.org/stream/IbnArabiTimeAndCosmology/Ibn-Arabi-Time-and-Cosmology_djvu.txt

Despite the fact that he considers time to be imagined and having no real existence, Ibn ‘Arabi stresses that it is one of the four ‘mothers (fundamental principles) of existence’: the formable monad (al-jawhar al-snwar f), 5 the acci- dental fonn (al- ‘arad), 6 time (al-zaman) and space (al-makan). 7 Everything else in the manifest world is combined of these four parameters [III.404.22]. He also 30 Ibn ‘Arabi’s concept of time argues that those four parameters - together with another six categories that are derived from them: fa'il, munfa'il, idafa, wad', ‘adad, kayf - are enough to describe the state of everything in the world. Together these make up the famil- iar ten Aristotelian categories: i.e. substance (jawhar), quantity ( kamm ), quality (kayf), relation (idafa), time (matd), place (ayna), situation or position ( wad ' ), possession (lahu), or state (Jidda), passion (yanfa'il ) and action (yaf'al ) - although the meaning of jawhar here is of course radically different from its usual Aristotelian usage, reflecting in this case the kalam inspiration of Ibn ‘Arabi’s terminology (El 2 , VI: 203, ‘Al-Makfdat’, and: EP, II: 46, ‘Categories’). Yet those four ‘mothers of existence’, including the formable monad, in Ibn ‘Arabi’s distinctive conception of the oneness of being, are nothing but imagi- nary forms or reflections of the unique ‘Single Monad’ (al-jawhar al-fard) which is the only thing that can be described as having a real existence: all other things in the world are different forms of this Single Monad, including ‘vision and the visible, hearing and the heard, imagination and the imaginable, thinking and the thinkable, . . . etc.’ [111.404.12]. This latter concept reflects Ibn ‘Arabi’s contro- versial theory of the oneness of being.

IBN ARABI FOUR TIME CYCLES

https://archive.org/stream/IbnArabiTimeAndCosmology/Ibn-Arabi-Time-and-Cosmology_djvu.txt

3.2 The four main time cycles Ibn ‘Arabi stresses that ‘everything in the world has to be based on (specific) divine Attributes’ [1.293.5]. Although some Muslim scholars, following a famous hadith [ Kanz : 1933, 1937], believe that the basic divine Names or Attributes of Allah can be limited to 99, Ibn ‘Arab! considers them to be count- less [III. 146.35], while the 99 Names that are referred to in some prophetic nar- rations are simply the main most Beautiful Names (al-asma ’ al-husna) of Allah. Of these many divine Names, there are four fundamental Attributes - Life ( havat ), Knowledge ( ‘Uni), Ability ( qudra ) and Will ( irada ) - that are necessary and sufficient for Allah to be described as God. Therefore those are considered to be the ultimate sources or ‘mothers’ ( ummahat ) of all other divine Attributes [1.469.25] . In relation to creation, however, three more Attributes are also neces- sary for Allah to be Creator: Hearing ( sam ' ), Seeing ( basar ) and Speaking (kalam). Together, that makes the principal divine Attributes of Allah to be ‘seven mother attributes. . . .: Life, Knowledge, Ability, Will, Hearing, Seeing and Speaking’ [1.525.32], Because Allah created (the perfect) Human Being ‘according to His Image’ [1.163.20], these same divine Attributes are potentially manifest in every fully human person (such as Adam and the prophets). Also, as Ibn ‘Arabi says, Allah created the world and everything in it in the image of (the Perfect) Human Being [11.652.25] , and so the world with the Human Being is ‘on the Image of the Real’ The significance of the divine week 77 - but without the Human Being it would not have this perfection [III.343.25]. So these same attributes should be available and essential in the world as well. That is why, he explains, the numbers four and seven play a central role in the world: the four elements in nature (earth, water, air and fire, already mentioned in his poem opening this chapter), the four time cycles, the seven heavens, the seven days, and so on. The two cosmologically fundamental four fold groups that emerged out of the four ‘mother’ Attributes (Life, Ability, Will and Power) that are the four aspects of the divine Presence of the Essence ( al-Dhat ) are the four earthly elements (earth, water, air and fire) and the primordial cosmological prin- ciples of the Intellect, Soul, Dust and Nature, as in Figure 3.1. This quadratic cosmological rule was also reflected in relation to time. There- fore, Ibn ‘Arabi points out, there are four main time cycles within the domain of manifest nature: the day, the week, the month and the year. These four natural time cycles have their origin in the effects of those four elements of Nature (fire, air, water, earth) that are originally derived from the above-mentioned four prin- cipal divine Names (‘the mothers’). As lbn ‘Arabi says: Figure 3. 1 The Divine Quadratic Rule. Note This figure is translated from the Futuhat [1.260], 78 The significance of the divine week time is restricted to the year, month, week and day. Time is divided into four divisions because the natural seasons are four, because the origin of the existence of time is Nature, whose level is below the (universal) Soul and above the ‘Dust’ ( haba ') that philosophers call the Universal Matter (. hayula ). The influence of this (principal) quaternity ( tarbV ) in Nature is from the influence of the (same principle of) quaternity in the divine influ- ences from (the fundamental Names) Life, Knowledge, Ability and Will. For by these four (Names), godship is confirmed for the God. So the quaternity (first) became manifest in Nature. Then the (divine) Command descended until the (principle of quaternity) appeared in the ‘biggest time’ (cycle), which is the year, so that it was divided into the four seasons: spring, summer, autumn and winter. This was brought about by the motion of the Sun through the stations (of the zodiac), which have been divided by Nature into their (seasonal) divisions according to the (natural) elements that are the ‘basic principles’ (of fire, air, water and earth). [chapter 390, III.548.17] 3.2.1 The day We have already discussed Ibn ‘Arabi’s concept of the ‘day’ in Chapter 2, where we showed that he defines the ‘day’ as all that is included within the revolution of the Isotropic Orb, which encompasses all of material existence. This day is astronomically defined by the rotation of the Earth and it is conventionally divided into smaller units such as hours, minutes and seconds (see also section 4.6). The divine Day, however, is the corresponding effects (manifestations) of each of the seven fundamental divine Names on the entire cosmos, as we shall see further below (section 3.4). This unique divine Day is in fact the smallest indivisible unit of time, though it equals in length the normal day as we dis- cussed in section 2.15. 3.2.2 The week The second time cycle is the week, which Ibn ‘Arab! - following the detailed Qur’anic indications - considers to be the main cycle of Creation. The week (which is seven days) has its origin in the seven main Attributes of Allah, but until now it does not seem to have any particular astronomical significance. However, Ibn ‘Arabi’s unique view, as explained below, gives a profound and essential significance to the Week in terms of astronomy/cosmology as well as the theology of creation. This will be the main focus throughout this chapter (see in particular section 3.3 below). 3.2.3 The month Ibn ‘Arabi distinguishes between the witnessed lunar month, which is from new moon to another new moon, and the ‘divine Month’ which is the time needed for The significance of the divine week 79 the Moon to perform one full revolution in the orb of the zodiac: that is, as Ibn ‘Arabi says, 28 days [III. 548. 28]. He also recognizes the solar ‘month’ as the Sun’s observed motion throughout the zodiac, where the zodiac is convention- ally divided into 12 parts, each corresponding to one month [1.388.20], though he does not give any details about the length of solar months in terms of their days. 10 3.2.4 The year The year, for Ibn ‘Arabi, is the time needed for the Sun to perform one full revo- lution in the orb of the zodiac [III. 548. 28], as witnessed from the Earth. Like the Babylonians, 11 Ibn ‘Arabi considers the year to be 360 days [III. 434. 9], and not like our calendar year of 365.25 days. Ibn ‘Arabi regards our solar year and the solar (and lunar) month as conven- tions set up by human observers, while the 360-day year, the 28-day month, the (seven-day) week/Week, and the (sidereal) day/Day are divine periods of time set up by Allah when He created the heavenly orbs and made them move [III. 548. 27]. It is noteworthy in this regard that the 360-day year does not equal 12 of the 28-day months. These four time cycles that Ibn ‘Arabi talks about are not meant for calendar purposes; they are said to be the actual measures of time set up by Allah when He created the world. Moreover, Ibn ‘Arabi shows that this non-integer ratio is preordained and essential for the vastness of creation, because the creation is built upon the act of generation ( takwin ), and with com- plete ratios no generation could happen; so there have to be integers and frac- tions [II. 440. 7]. The differences between the witnessed lunar month (synodic lunar month = 29.53 days) and the divine lunar month (of 28 days), and between the witnessed year (365.25 days) and the 360-day year, might be because of the interference of the different motions of the Sun, the Earth and the Moon. As the Earth spins around its axis it also rotates around the Sun, and as the Moon rotates around the Earth it also moves with the Earth around the Sun. These interfering motions may account for the difference. For example, if we measure the period of the Moon relative to those stars that are apparently fixed (this is called the sidereal lunar month), we get only 27.32 days (and not the usual 29.53 days, the observed lunar month). The divine lunar month for Ibn ‘Arabi is 28 days because he meas- ures that period in relation to the zodiac (far-away galaxies) or actually the Iso- tropic Orb, and not the orb of the Sun or the constellations [1.656.13], because those constellations are not actually fixed [III. 549. 3]. Also we have to know that the length of the observed earthly day varies from one place to another on the Earth and from summer to winter throughout the year, and that the normal solar year and the normal lunar month slightly vary from time to time owing to the influence of gravitation of other planets and stars that change their positions inside and relative to the solar system, respectively. Thus the mean solar day in the year 2000 is about 1.7 milliseconds longer than it was in 1900, and is slowly getting longer. There is a possible allusion in the Qur’an to such long-term 80 The significance of the divine week changes in the length of the year and the month, where Allah says: ‘ The quantity of months with Allah is 12 months (in a year) by Allah ’s ordinance in the day that (when) He created the Heavens and the Earth ’ (9:36): in other words, it could be that the year started as 12 months each of 28 days, but that this then changed with time as the motion of the Earth slowed down and as the solar year became longer, and also as the lunar month became longer than 28 days. In this regard, we should also notice that in Arabic there are two names for the year which do not appear to have identical meanings: sana and ‘am. Although both terms are currently used to refer to the year, it seems from the etymological meaning of those two names and from Ibn ‘Arabi’s and Qur’anic usage that the word sana means the original 360-day year, while ‘am - which literally means ‘entire’ or ‘full’ - is the time needed for the Earth to make a full revolution around the Sun, which is the slowly lengthening conventional year now observed on Earth. In the Qur’an, Allah distinguishes between these two Arabic words in one verse that declares the time that Prophet Noah stayed with his folk: ‘ And verily We sent Noah unto his folk, and he continued with them for a thousand years (sana) save fifty years (‘am); and the flood engulfed them, for they were wrong doers ’ (29: 14). 3.3 The week as the primary time cycle While lbn ‘Arab! considers the Week (of Creation) to be the primary time cycle, only the week among these four cycles does not seem to have any apparent astronomical significance. We can say only that the week is one-quarter of the divine lunar month (28 = 4x7). From the observed astronomical point of view, the day should be the primary time cycle, because it is the smallest standard period of time as far as the solar system and the Earth are concerned, and all other three cycles (as defined by Ibn ‘Arabi) are integer multiples of the day, while the year is not an integer multiple of the week. However, we shall see that lbn ‘Arabi does not consider the day to be the primary cycle because the Days of the divine Week are not similar to each other, as they might appear to us. Since each Day of the Week is based on one of the seven fundamental divine Attributes of Allah, so these Days are not identical because those seven divine Attributes are not identical. Therefore the Week, rather than the day, is the primary cycle of divine time, and each day of the seven Days of that Week is ruled by one of the seven fundamental divine Attributes. However, in keeping with lbn ‘Arabi’s essential understanding of the ‘ever- new creation’, this does not mean that any particular day of this week is identical to that of another week. They are only ‘similar’ to each other because they are originated from the same divine Attribute. Ibn ‘Arabi says: Nothing is actually repeated, because of divine vastness ( ittisa ); so (every- thing) is in ever-new, not renewed, existence. Thus if we call the new (thing) ‘renewed’, that is because it is extremely similar (but not identical) to its counterpart, so that they can not be distinguished from each other . . . and The significance of the divine week 8 1 the daytime and night are called ‘the two-new’ ( al-jadidan ), and not ‘the two-renewed’ ( al-mutajaddidan ), because Saturday is not Sunday and it is not Saturday from the other week, or from another month or from another year. [III. 127.23] This is clearly evident in modem astronomy, because whatever periodical motions we see locally in our solar system are actually part of a more global motion that, in the end, never repeats itself in the same way, because everything is moving (see sections 1.1 and 1.4). In fact, Ibn ‘Arab! always stresses that there can not be any two identical forms in the world, and that this is because ‘Allah never manifests in the same form twice, nor in the same form to any two persons’ [III. 127.33], Therefore Ibn ‘Arab! maintains that ‘although there are many days, the real order of events reduces them into seven days’ [Ayyam Al-Sha’n : 6], which are the seven days of the week; and then these days iterate in months and years. And as we showed, this is due to the fact that ‘(the main) divine Attributes are seven, not more, which made the Age not more than seven (distinctive) Days’ [11.437.30],

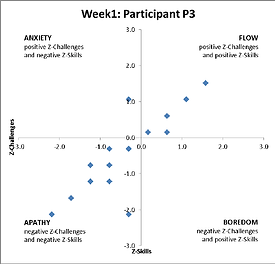
https://www.researchgate.net/figure/266899110_fig17_FIGURE-53-THE-QUADRANT-MODEL-OF-FLOW-GRAPHICAL-REPRESENTATION-OF-THE-Z-SCORES-OF

https://www.researchgate.net/profile/Pratyush_Pandab2/publication/266899110/figure/fig17/AS:295661154127875@1447502520498/FIGURE-53-THE-QUADRANT-MODEL-OF-FLOW-GRAPHICAL-REPRESENTATION-OF-THE-Z-SCORES-OF.png

Flow is described as an autotelic experience that is poised between boredom and anxiety (section 2.3). Based on this theoretical definition of flow and using The Quadrant Model (refer The Quadrant Model ) for measurement of flow conditions, the percentage-of-time in flow was calculated (section 3.2.1). First , each participant’s vector of raw scores of challenge and skill was individually standardized with z- scores using SPSS. These resultant scores (z-skills and z-challenges) were then divided into one of the four quadrants depending on whether challenges and skills were above the individual’s average (i.e. have positive Z-scores) or not (section 3.2.1). FIGURE 5.3 shows the graphical representation of z-skills and z- challenges for Week1 on z-skills and z-challenges Cartesian plane for participant P3. Plotting of the z- scores onto the Cartesian plane was a many-to-one mapping, as multiple z-scores had same values. For instance, in the data set shown in FIGURE 5.3 the z-skills and z-challenges set (1.6, 1.5) (highlighted) occurs more than once, but is represented as a single point on the Cartesian plane. Hence, along with a graphical representation, a manual check was done to count the total number of flow states. FIGURE 5.4 shows the result after the manual check was performed for participant P3 for Week1. A similar manual check procedure was followed for each of the participants for their data sets for all the three weeks. FIGURE 5.5 shows the graphical representation of the data set and the manual check result for Week1 for participant P5.

THE QUADRANT MODEL OF FLOW: GRAPHICAL REPRESENTATION OF THE Z-SCORES OF CHALLENGES AND SKILLS OF PARTICIPANT P3 FOR WEEK1 (LEFT), AND RAW SCORES AND Z-SCORES OF CHALLENGES AND SKILLS OF PARTICIPANT P3 FOR WEEK1

FOUR QUADRANTS FLOW

https://mba.midlandu.edu/blog/%22Flow:%20The%20Psychology%20of%20Optimal%20Experience%22

Csikszentmihalyi talks about the importance of intrinsic motivation. People who achieve a state of flow are most often working on projects that they care deeply about. They are achieving the tasks simply because they are important to them–not because they are responding to pressure from others.

However, the bulk of his theory deals with how your skill level and the relative challenge of a task or project will change your mental state. You can roughly envision the flow model as a grid with four quadrants based on the person's skill and the level of the challenge of the task at hand. (Csikszentmihalyi covers nine states, but we'll concentrate on these four).

Apathy: Low Skill / Low Challenge: When a person is not skilled at a task, but the challenge-level of the task is low as well, this quadrant is described as “Apathy.” The person asked to do the project will have little motivation and will devote little mental ability to completing the job.

Anxiety: Low Skill / High Challenge: In situations where a task is difficult, but the person has little skill in the area, the person may become anxious or nervous about a job.

Relaxation: High Skill / Low Challenge: Where a person has a lot of experience, and is taking on a job that is simple based on their level of experience, the tasks can create a sense of ease. Note that in this state, engagement may not be high, because the job can be fairly routine.

Flow: High Skill / High Challenge: This is the state most likely to create the optimal mix of focus and intensity. Working on these types of tasks will get someone highly engaged in their job.

Understanding the concepts in the book can help business professionals in a couple of ways. It’s essential to be aware of what state each task is likely to inspire in you based on the challenge/skill matrix. If a project or task is sitting in the Apathy quadrant, you're going to need to manage your time and focus much more tightly to accomplish the task. Conversely, when you're not “feeling it,” switch to tasks that sit in the Flow quadrant in order to get yourself moving.

In addition, understanding these quadrants can help managers assign and distribute projects to their staff. There's a temptation to give more junior members of the team the “simpler” tasks. However, be sure that you're managing workload to maximize the engagement of all employees. Look at all the tasks and contemplate where they sit on the grid for your team.

FOUR STRINGS AND FOUR COURSES OF STRINGS

https://en.wikipedia.org/wiki/Four-string_guitar

Guitar family instruments with four strings[edit]

Bass guitar

Braguinha

Cak used in Kroncong music

Cavaquinho

Celovic used in Tamburica orchestras

Cuatro Venezolano

Tenor guitar

Ukulele

Cigar box guitars often have three or four strings.

Guitar family instruments with four courses of strings[edit]

The Renaissance guitar.

Brac and Bugaria used in Tamburica orchestras

Some Chitarra Battentes

Some Kabosys

Tahitian ukulele

Tiple

FOUR STRING

https://en.wikipedia.org/wiki/Cuatro_(Venezuela)

The cuatro of Venezuela has four single nylon strings, tuned (ad'f#'b). It is similar in shape and tuning to the ukulele, but their character and playing technique are vastly different. It is tuned in a similar fashion to the traditional D tuning of the ukulele, but the A and B are an octave lower. Consequently, the same fingering can be used to shape the chords, but it produces a different inversion of each chord.[1] A cuatro player is called a cuatrista.

Its 15th-Century ancestor was the Portuguese Cavaquinho. The predecessor of the Venezuelan cuatro is the four-string Spanish guitar which disappeared in the 16th century after a short period of surging popularity. In the 1950s, Fredy Reyna documented the evolution of the renaissance guitar into the current Venezuelan Cuatro, and reinvented the cuatro as a solo instrument, equally capable of rendering traditional Venezuelan music as well as Renaissance pieces. The popularity of the instrument in Venezuela and elsewhere may be due to its apparent simplicity, having only four strings, as well as its compact size.

A popular way to remember the tuning of the cuatro among Venezuelan cuatro players is to play each string individually from top to bottom, while singing the words "Cam-bur pin-tón" in the same expected notes of the four cuatro strings. (Cambur Pintón means Ripe Banana in Venezuela. The phrase is used mainly because its four syllables are long and because of its easy-to-remember nature). A variation is "Hi-pó-cri-ta", playing the strings from bottom to top.

WALT WHITMAN ON THE QUATERNITY

http://elizabethr-pphs2011.blogspot.com/2012/04/chanting-square-deific.html

"Chanting the Square Deific"

Walt Whitman's "Chanting the Square Deific" interprets a different spin on the classic Christian Holy Trinity. Instead of a trinity, Whitman writes of a "quaternity" of figures (Oliver). The first side of the square is God, but not just the Christian God. Whitman's first side of the "Square Deific" is the God of Hebrews, "Jehovah"; God of Hindu religion, "Brahm"; God of Romans, "Saturn", and God of Greeks, "Kronos" (Whitman). The main thing that the gods have in common is that they represent the leader or chief of their respective religious deities, just like God of Christianity's Holy Trinity is the top of the triangle. As opposed the the Christian God, the first side of the "Square Deific" is not merciless as He "forgives no man" and "lets none expect mercy" (Whitman). That is a major difference separating the Holy Trinity and the Square Deific. The first stanza exhibits the power of the first deity. The God decides "judgments without the least remorse" and has all of His subjects and followers' lives in His hands (Whitman). The extent of His power is shown when the seasons and Earth's gravitation pull are mentioned, as the Square Deity is as constant, reliable, and relentless as the aforementioned acts of nature (Whitman).

The second stanza describes the next side of the Square, the son of the first side. The knowledge that the second side deity is the offspring of the first comes from the mythological references of the "Lord Jesus", "Hercules", and "Hermes" (Whitman). Jesus was the next part of the Christian Holy Trinity, which seems to be a model for the Square Deific. Hercules was the son of Zeus, another chief deity and rose to power and eventually became a god himself. Hermes was another son of Zeus, ruler of Greek Gods. The Square Deific second side diety represents the people better than the first deity as he "absorbs the suffering", "crucified", "taunted", "cheer bringer", and His "charity has no death" (Whitman). That signifies that His influence and support will always be present in whatever way is desired and can bring comfort to the people that He is there (Oliver).

The third stanza, the third side of the Square, is the opposite of the deity representing the first side. In Christianity the Devil is the opposite of God, as well as evil is the opposite of goodness. But the "Square Deific" version of Satan is not as evil as the Christian version. The Square Deity is the "brother to the slaves" and is not necessarily evil (Whitman). The deity is stubborn and will live up to his "permanent" vows and decisions (Whitman). That makes Him a respectable God as one can always trust him to keep his word, but with the knowledge that He is not looking out for their benefit as well as the second side of the Square Deific will be.

The final side of the Square is the equivalent of the last part of the Holy Trinity. The Holy Spirit in Christianity represents the invisible acts from God that help His followers better understand their religion and Him. The "Santa Spirita" is the "breather of life" which is very similar to the Holy Spirit, but the Spirit is the most "solid" part of the square, therefore the most important part (Whitman). The Spirit triumphs over Heaven and Hell ( "lighter than light", "flames of hell", "Paradise" ) and "including God, the Saviour, and the Satan" in Its rank (Whitman). The last line of the poem makes the reader assume that the narrator, Walt Whitman, either is the "Santa Spirita" or has the "Santa Spirita" within him, which is another trait of the Christian Holy Spirt (Oliver). Whitman's "Square Deific" is a well rounded Holy Trinity, with all sides of humanity represented in good and evil as well as self and savior. Whitman expanded on the classic Trinity, but improved it to represent what he envisioned as the deities of man.

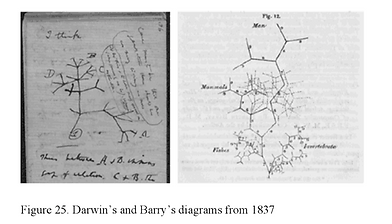
DARWIN ADDS A FOURTH BRAHNCH

https://books.google.com/books?id=ESFo2r9CvtIC&pg=PT122&lpg=PT122&dq=von+Baer+four+archetypes&source=bl&ots=TlK6g3X1lb&sig=Dautig629XcATk91EYlueqx6CZs&hl=en&sa=X&ved=0ahUKEwivkvDCvPHTAhUN9GMKHdy7B5UQ6AEITDAH#v=snippet&q=four&f=false

DARWINS FOUR FINCHES

https://en.wikipedia.org/wiki/File:Finchadaptiveradiation.png

https://en.wikipedia.org/wiki/Darwin%27s_finches

At the time that he rewrote his diary for publication as Journal and Remarks (later The Voyage of the Beagle), he described Gould's findings on the number of birds, noting that "Although the species are thus peculiar to the archipelago, yet nearly all in their general structure, habits, colour of feathers, and even tone of voice, are strictly American".[19] In the first edition of The Voyage of the Beagle, Darwin said that "It is very remarkable that a nearly perfect gradation of structure in this one group can be traced in the form of the beak, from one exceeding in dimensions that of the largest gros-beak, to another differing but little from that of a warbler".[20]

By the time the first edition was published, the development of Darwin's theory of natural selection was in progress. For the 1845 second edition of The Voyage (now titled Journal of Researches), Darwin added more detail about the beaks of the birds, and two closing sentences which reflected his changed ideas: "Seeing this gradation and diversity of structure in one small, intimately related group of birds, one might really fancy that from an original paucity of birds in this archipelago, one species had been taken and modified for different ends."[21][22]

The remaining land-birds form a most singular group of finches, related to each other in the structure of their beaks, short tails, form of body and plumage: there are thirteen species, which Mr. Gould has divided into four subgroups. All these species are peculiar to this archipelago; and so is the whole group, with the exception of one species of the sub-group Cactornis, lately brought from Bow Island, in the Low Archipelago. Of Cactornis, the two species may be often seen climbing about the flowers of the great cactus-trees; but all the other species of this group of finches, mingled together in flocks, feed on the dry and sterile ground of the lower districts. The males of all, or certainly of the greater number, are jet black; and the females (with perhaps one or two exceptions) are brown. The most curious fact is the perfect gradation in the size of the beaks in the different species of Geospiza, from one as large as that of a hawfinch to that of a chaffinch, and (if Mr. Gould is right in including his sub-group, Certhidea, in the main group) even to that of a warbler. The largest beak in the genus Geospiza is shown in Fig. 1, and the smallest in Fig. 3; but instead of there being only one intermediate species, with a beak of the size shown in Fig. 2, there are no less than six species with insensibly graduated beaks. The beak of the sub-group Certhidea, is shown in Fig. 4. The beak of Cactornis is somewhat like that of a starling, and that of the fourth subgroup, Camarhynchus, is slightly parrot-shaped. Seeing this gradation and diversity of structure in one small, intimately related group of birds, one might really fancy that from an original paucity of birds in this archipelago, one species had been taken and modified for different ends. In a like manner it might be fancied that a bird originally a buzzard, had been induced here to undertake the office of the carrion-feeding Polybori of the American continent.[23]

Seen here is adapted radiation of finch A. (Geospiza magnirostris) into three other species of finches found on the Galapagos Islands. Due to the absence of other species of birds, the finches adapted to new niches. The finches beaks and bodies changed allowing them to eat certain types of foods such as nuts, fruits, and insects.

Geospiza magnirostris

Geospiza parvula

Certhidea olivacea

Geospiza fortis

Large ground finch, Medium ground finch
Small tree finch, Green warbler-finch

PICASSOS GUERNICA AND THE CRUCIFORM FIGURE AND THE PIETA

http://yaledailynews.com/blog/2003/04/04/art-and-war-jay-winter/

To be sure, on the left hand side of the painting is a pieta; the mother grieving over the child is palpable. Below this ensemble and to the right is the cruciform figure of the dead male figure, with a broken sword and severed arm on one side an outstretched hand on the other.

https://www.pablopicasso.org/crucifixion.jsp

HAMILTON AND QUATERNIONS AND THE QUATERNION PLAQUE

https://en.wikipedia.org/wiki/File:William_Rowan_Hamilton_Plaque_-_geograph.org.uk_-_347941.jpg

Quaternions[edit]

https://en.wikipedia.org/wiki/William_Rowan_Hamilton

Quaternion Plaque on Broom Bridge

Main article: History of quaternions

The other great contribution Hamilton made to mathematical science was his discovery of quaternions in 1843. However, in 1840, Benjamin Olinde Rodrigues had already reached a result that amounted to their discovery in all but name.[9]

Hamilton was looking for ways of extending complex numbers (which can be viewed as points on a 2-dimensional plane) to higher spatial dimensions. He failed to find a useful 3-dimensional system (in modern terminology, he failed to find a real, three-dimensional skew-field), but in working with four dimensions he created quaternions. According to Hamilton, on 16 October he was out walking along the Royal Canal in Dublin with his wife when the solution in the form of the equation

i2 = j2 = k2 = ijk = −1

suddenly occurred to him; Hamilton then promptly carved this equation using his penknife into the side of the nearby Broom Bridge (which Hamilton called Brougham Bridge). This event marks the discovery of the quaternion group.

A plaque under the bridge was unveiled by the Taoiseach Éamon de Valera, himself a mathematician and student of quaternions,[10] on 13 November 1958.[11]

Since 1989, the National University of Ireland, Maynooth has organised a pilgrimage, where mathematicians take a walk from Dunsink Observatory to the bridge, where no trace of the carving remains, though a stone plaque does commemorate the discovery.[12]

The quaternion involved abandoning commutativity, a radical step for the time. Not only this, but Hamilton had in a sense invented the cross and dot products of vector algebra. Hamilton also described a quaternion as an ordered four-element multiple of real numbers, and described the first element as the 'scalar' part, and the remaining three as the 'vector' part.

Hamilton introduced, as a method of analysis, both quaternions and biquaternions, the extension to eight dimensions by introduction of complex number coefficients. When his work was assembled in 1853, the book Lectures on Quaternions had "formed the subject of successive courses of lectures, delivered in 1848 and subsequent years, in the Halls of Trinity College, Dublin". Hamilton confidently declared that quaternions would be found to have a powerful influence as an instrument of research. When he died, Hamilton was working on a definitive statement of quaternion science. His son William Edwin Hamilton brought the Elements of Quaternions, a hefty volume of 762 pages, to publication in 1866. As copies ran short, a second edition was prepared by Charles Jasper Joly, when the book was split into two volumes, the first appearing 1899 and the second in 1901. The subject index and footnotes in this second edition improved the Elements accessibility.

One of the features of Hamilton's quaternion system was the differential operator del which could be used to express the gradient of a vector field or to express the curl. These operations were applied by Clerk Maxwell to the electrical and magnetic studies of Michael Faraday in Maxwell's Treatise on Electricity and Magnetism (1873). Though the del operator continues to be used, the real quaternions fall short as a representation of spacetime. On the other hand, the biquaternion algebra, in the hands of Arthur W. Conway and Ludwik Silberstein, provided representational tools for Minkowski space and the Lorentz group early in the twentieth century.

Today, the quaternions are used in computer graphics, control theory, signal processing, and orbital mechanics, mainly for representing rotations/orientations. For example, it is common for spacecraft attitude-control systems to be commanded in terms of quaternions, which are also used to telemeter their current attitude. The rationale is that combining quaternion transformations is more numerically stable than combining many matrix transformations. In control and modelling applications, quaternions do not have a computational singularity (undefined division by zero) that can occur for quarter-turn rotations (90 degrees) that are achievable by many Air, Sea and Space vehicles. In pure mathematics, quaternions show up significantly as one of the four finite-dimensional normed division algebras over the real numbers, with applications throughout algebra and geometry.

"Time is said to have only one dimension, and space to have three dimensions. ... The mathematical quaternion partakes of both these elements; in technical language it may be said to be 'time plus space', or 'space plus time': and in this sense it has, or at least involves a reference to, four dimensions. And how the One of Time, of Space the Three, Might in the Chain of Symbols girdled be."—William Rowan Hamilton (quoted in Robert Percival Graves' "Life of Sir William Rowan Hamilton" (3 volumes, 1882, 1885, 1889))

William Rowan Hamilton's scientific career included the study of geometrical optics, classical mechanics, adaptation of dynamic methods in optical systems, applying quaternion and vector methods to problems in mechanics and in geometry, development of theories of conjugate algebraic couple functions (in which complex numbers are constructed as ordered pairs of real numbers), solvability of polynomial equations and general quintic polynomial solvable by radicals, the analysis on Fluctuating Functions (and the ideas from Fourier analysis), linear operators on quaternions and proving a result for linear operators on the space of quaternions (which is a special case of the general theorem which today is known as the Cayley–Hamilton theorem). Hamilton also invented "icosian calculus", which he used to investigate closed edge paths on a dodecahedron that visit each vertex exactly once.

QUATERNIONS- THERE ARE FOUR NORMED ALGEBRAS ONE OF WHICH IS THE QUATERNION

https://en.wikipedia.org/wiki/History_of_quaternions

In mathematics, quaternions are a non-commutative number system that extends the complex numbers. Quaternions and their applications to rotations were first described in print by Olinde Rodrigues in all but name in 1840, but independently discovered by Irish mathematician Sir William Rowan Hamilton in 1843 and applied to mechanics in three-dimensional space. They find uses in both theoretical and applied mathematics, in particular for calculations involving three-dimensional rotations. This article describes the original invention and subsequent development of quaternions.

In 1843, Hamilton knew that the complex numbers could be viewed as points in a plane and that they could be added and multiplied together using certain geometric operations. Hamilton sought to find a way to do the same for points in space. Points in space can be represented by their coordinates, which are triples of numbers and have an obvious addition, but Hamilton had difficulty defining the appropriate multiplication.

According to a letter Hamilton wrote later to his son Archibald:

Every morning in the early part of October 1843, on my coming down to breakfast, your brother William Edward and yourself used to ask me: "Well, Papa, can you multiply triples?" Whereto I was always obliged to reply, with a sad shake of the head, "No, I can only add and subtract them."

On October 16, 1843, Hamilton and his wife took a walk along the Royal Canal in Dublin. While they walked across Brougham Bridge (now Broom Bridge), a solution suddenly occurred to him. While he could not "multiply triples", he saw a way to do so for quadruples. By using three of the numbers in the quadruple as the points of a coordinate in space, Hamilton could represent points in space by his new system of numbers. He then carved the basic rules for multiplication into the bridge:

−

i^{2}=j^{2}=k^{2}=ijk=-1.\,

Hamilton called a quadruple with these rules of multiplication a quaternion, and he devoted the remainder of his life to studying and teaching them. From 1844 to 1850 Philosophical Magazine communicated Hamilton's exposition of quaternions.[1] In 1853 he issued Lectures on Quaternions, a comprehensive treatise that also described biquaternions. The facility of the algebra in expressing geometric relationships led to broad acceptance of the method, several compositions by other authors, and stimulation of applied algebra generally. As mathematical terminology has grown since that time, and usage of some terms has changed, the traditional expressions are referred to classical Hamiltonian quaternions.

Hamilton's innovation consisted of expressing quaternions as an algebra over R. The formulae for the multiplication of quaternions are implicit in the four squares formula devised by Leonhard Euler in 1748; Olinde Rodrigues applied this formula to representing rotations in 1840.[2]:9

Response[edit]

The special claims of quaternions as the algebra of four-dimensional space were challenged by James Cockle with his exhibits in 1848 and 1849 of tessarines and coquaternions as alternatives. Nevertheless, these new algebras from Cockle were, in fact, to be found inside Hamilton’s biquaternions. From Italy, in 1858 Giusto Bellavitis responded[3] to connect Hamilton’s vector theory with his theory of equipollences of directed line segments.

Jules Hoüel led the response from France in 1874 with a textbook on the elements of quaternions. To ease the study of versors, he introduced "biradials" to designate great circle arcs on the sphere. Then the quaternion algebra provided the foundation for spherical trigonometry introduced in chapter 9. Hoüel replaced Hamilton’s basis vectors i,j,k with i1, i2, and i3. The variety of typefaces (fonts) available led Hoüel to another notational innovation: A designates a point, a and

{\mathrm {a}} are algebraic quantities, and in the equation for a quaternion

cos

⁡

sin

⁡

{\mathcal {A}}=\cos \alpha +{\mathbf {A}}\sin \alpha ,

\mathbf {A} is a vector and α is an angle. This style of quaternion exposition was perpetuated by Charles-Ange Laisant[4] and Alexander Macfarlane.[5]

William K. Clifford expanded the types of biquaternions, and explored elliptic space, a geometry in which the points can be viewed as versors. Fascination with quaternions began before the language of set theory and mathematical structures was available. In fact, there was little mathematical notation before the Formulario mathematico. The quaternions stimulated these advances: For example, the idea of a vector space borrowed Hamilton’s term but changed its meaning. Under the modern understanding, any quaternion is a vector in four-dimensional space. (Hamilton’s vectors lie in the subspace with scalar part zero.)

Since quaternions demand their readers to imagine four dimensions, there is a metaphysical aspect to their invocation. Quaternions are a philosophical object. Setting quaternions before freshmen students of engineering asks too much. Yet the utility of dot products and cross products in three-dimensional space, for illustration of processes, calls for the uses of these operations which are cut out of the quaternion product. Thus Willard Gibbs and Oliver Heaviside made this accommodation, for pragmatism, to avoid the distracting superstructure.[6]

For mathematicians the quaternion structure became familiar and lost its status as something mathematically interesting. Thus in England, when Buchheim prepared a paper on biquaternions, it was published in the American Journal of Mathematics since some novelty in the subject lingered there. Research turned to hypercomplex numbers more generally. For instance, Thomas Kirkman and Arthur Cayley considered the number of equations between basis vectors would be necessary to determine a unique system. The wide interest that quaternions aroused around the world resulted in the Quaternion Society. In contemporary mathematics, the division ring of quaternions exemplifies an algebra over a field.

DESCRIBED BY FOUR PARAMETERS

https://en.wikipedia.org/wiki/Euler–Rodrigues_formula

In mathematics and mechanics, the Euler–Rodrigues formula describes the rotation of a vector in three dimensions. It is based on Rodrigues' rotation formula, but uses a different parametrization.

The rotation is described by four Euler parameters due to Leonhard Euler. The Rodrigues formula (named after Olinde Rodrigues), a method of calculating the position of a rotated point, is used in some software applications, such as flight simulators and computer games.

A rotation about the origin is represented by four real numbers, a, b, c, d such that

a^{2}+b^{2}+c^{2}+d^{2}=1.

When the rotation is applied, a point at position x→ rotates to its new position

→

′

(

−

2(bc−ad) 2(bd+ac) 2(bc+ad)

−

2(cd−ab) 2(bd−ac) 2(cd+ab)

−

)

→

{\vec x}'={\begin{pmatrix}a^{2}+b^{2}-c^{2}-d^{2}&2(bc-ad)&2(bd+ac)\\2(bc+ad)&a^{2}+c^{2}-b^{2}-d^{2}&2(cd-ab)\\2(bd-ac)&2(cd+ab)&a^{2}+d^{2}-b^{2}-c^{2}\end{pmatrix}}{\vec x}.

The parameters (a, b, c, d) and (−a, −b, −c, −d) describe the same rotation. Apart from this symmetry, every set of four parameters describes a unique rotation in three-dimensional space.

Composition of rotations[edit]

The composition of two rotations is itself a rotation. Let (a1, b1, c1, d1) and (a2, b2, c2, d2) be the Euler parameters of two rotations. The parameters for the compound rotation (rotation 2 after rotation 1) are as follows:

a =

−

; b =

−

; c =

−

; d =

−

.{\begin{aligned}a&=a_{1}a_{2}-b_{1}b_{2}-c_{1}c_{2}-d_{1}d_{2};\\b&=a_{1}b_{2}+b_{1}a_{2}-c_{1}d_{2}+d_{1}c_{2};\\c&=a_{1}c_{2}+c_{1}a_{2}-d_{1}b_{2}+b_{1}d_{2};\\d&=a_{1}d_{2}+d_{1}a_{2}-b_{1}c_{2}+c_{1}b_{2}.\end{aligned}}

It is straightforward, though tedious, to check that a2 + b2 + c2 + d2 = 1. (This is essentially Euler's four-square identity, also used by Rodrigues.)

TWO BY TWO MATRICES ALSO CALLED PAULI MATRICES- TWO BY TWO IS A QUADRANT- BIQUATERNIONS

https://en.wikipedia.org/wiki/Spinors_in_three_dimensions

The association of a spinor with a 2×2 complex Hermitian matrix was formulated by Élie Cartan.[1]

In detail, given a vector x = (x1, x2, x3) of real (or complex) numbers, one can associate the complex matrix

→

(

−i

−

)

{\displaystyle {\vec {x}}\rightarrow X\ =\left({\begin{matrix}x_{3}&x_{1}-ix_{2}\\x_{1}+ix_{2}&-x_{3}\end{matrix}}\right).}

Matrices of this form have the following properties, which relate them intrinsically to the geometry of 3-space:

det X = – (length x)2.

X 2 = (length x)2I, where I is the identity matrix.

(

)

(

⋅

)

\frac{1}{2}(XY+YX)=({\bold x}\cdot{\bold y})I [1]:43

(

−

)

\frac{1}{2}(XY-YX)=iZ where Z is the matrix associated to the cross product z = x × y.

If u is a unit vector, then −UXU is the matrix associated to the vector obtained from x by reflection in the plane orthogonal to u.

It is an elementary fact from linear algebra that any rotation in 3-space factors as a composition of two reflections. (Similarly, any orientation reversing orthogonal transformation is either a reflection or the product of three reflections.) Thus if R is a rotation, decomposing as the reflection in the plane perpendicular to a unit vector u1 followed by the plane perpendicular to u2, then the matrix U2U1XU1U2 represents the rotation of the vector x through R.

Having effectively encoded all of the rotational linear geometry of 3-space into a set of complex 2×2 matrices, it is natural to ask what role, if any, the 2×1 matrices (i.e., the column vectors) play. Provisionally, a spinor is a column vector

[

]

\xi=\left[\begin{matrix}\xi_1\\\xi_2\end{matrix}\right], with complex entries ξ1 and ξ2.

The space of spinors is evidently acted upon by complex 2×2 matrices. Furthermore, the product of two reflections in a given pair of unit vectors defines a 2×2 matrix whose action on euclidean vectors is a rotation, so there is an action of rotations on spinors. However, there is one important caveat: the factorization of a rotation is not unique. Clearly, if X → RXR−1 is a representation of a rotation, then replacing R by −R will yield the same rotation. In fact, one can easily show that this is the only ambiguity which arises. Thus the action of a rotation on a spinor is always double-valued.

There were some precursors to Cartan's work with 2×2 complex matrices: Wolfgang Pauli had used these matrices so intently that elements of a certain basis of a four-dimensional subspace are called Pauli matrices σi, so that the Hermitian matrix is written as a Pauli vector

→

⋅

→

{\displaystyle {\vec {x}}\cdot {\vec {\sigma }}.}[2] In the mid 19th century the algebraic operations of this algebra of four complex dimensions were studied as biquaternions.

Often, the first example of spinors that a student of physics encounters are the 2×1 spinors used in Pauli's theory of electron spin. The Pauli matrices are a vector of three 2×2 matrices that are used as spin operators.

THE FOUR FAMILIES OF LIE ALGEBRAS

https://en.wikipedia.org/wiki/Semisimple_Lie_algebra

As explained in greater detail below, semisimple Lie algebras over

\mathbb {C} are classified by the root system associated to their Cartan subalgebras, and the root systems, in turn, are classified by their Dynkin diagrams. Examples of semisimple Lie algebras, with notation coming from their Dynkin diagrams, are:

A_{n}:

{\mathfrak {sl}}_{{n+1}}, the special linear Lie algebra.

B_{n}:

{\mathfrak {so}}_{{2n+1}}, the odd-dimensional special orthogonal Lie algebra.

C_{n}:

{\mathfrak {sp}}_{{2n}}, the symplectic Lie algebra.

D_{n}:

{\mathfrak {so}}_{{2n}}, the even-dimensional special orthogonal Lie algebra.

These Lie algebras are numbered so that n is the rank. Except certain exceptions in low dimensions, many of these are simple Lie algebras, which are a fortiori semisimple. These four families, together with five exceptions (E6, E7, E8, F4, and G2), are in fact the only simple Lie algebras over the complex numbers.

Every semisimple Lie algebra over an algebraically closed field of characteristic 0 is a direct sum of simple Lie algebras (by definition), and the finite-dimensional simple Lie algebras fall in four families – An, Bn, Cn, and Dn – with five exceptions E6, E7, E8, F4, and G2. Simple Lie algebras are classified by the connected Dynkin diagrams, shown on the right, while semisimple Lie algebras correspond to not necessarily connected Dynkin diagrams, where each component of the diagram corresponds to a summand of the decomposition of the semisimple Lie algebra into simple Lie algebras.

for Dn. If one starts numbering lower, the enumeration is redundant, and one has exceptional isomorphisms between simple Lie algebras, which are reflected in isomorphisms of Dynkin diagrams; the En can also be extended down, but below E6 are isomorphic to other, non-exceptional algebras.

1,2,4, AND 8 RHCO ARE THE ONLY NORMED ALGEBRAS

2 Jordan Algebras in the Algebraic Renaissance: Finite ... - Springer

www.springer.com/cda/content/document/cda.../9780387954479-c1.pdf?SGWID...

Elie Cartan in the 1890s, they were known only through their multiplication ..... In 1748 Euler used an extension of this to “quaternary” (4-variable) quadratic.

The algebra behind the 2-square formula is just the complex numbers C : z = x01+x1i with basis 1, i over the reals, where 1 acts as identity and i2 = −1 and N(z) = x20 + x21 = zz is the ordinary norm squared (where z = x01 − x1i is the ordinary complex conjugate). This interpretation was well known to Gauss. The 4-squares formula led Hamilton to the quaternions H consisting of all x = x01+x1i+x2j +x3k, where the formula for x·y means that the basis elements 1, i, j, k satisfy the now-familiar rules

i2 =j2 =k2 =−1, ij=−ji=k, jk=−kj=i, ki=−ik=j.

Clearly, this algebra is no longer commutative. Again N(x) = xx is the ordi- nary norm squared (where x = x01−x1i−x2j−x3k is the ordinary quaternion conjugate).

Clifford and Hamilton invented 8-dimensional algebras (biquaternions), which were merely the direct sum H H of two quaternion algebras. Be- cause of the presence of zero divisors, these algebras were of minor in- terest. Cayley was the first to use the 8-square formula to create an 8- dimensional division algebra K of octonions or Cayley numbers. By 1847 he

2.10 Composition Algebras 63

recognized that this algebra was not commutative or associative, with basis e0,... ,e7 = 1,i,j,k,l,il,jl,kl with multiplication table

e0ei =eie0 =ei, e2i =−1, eiej =−ejei =ek for ijk = 123, 145, 624, 653, 725, 734, 176.

A subsequent flood of (false!!) higher-dimensional algebras carried names such as quadrinions, quines, pluquaternions, nonions, tettarions, plutonions. Ire- land especially seemed a factory for such counterfeit division algebras. In 1878 Frobenius showed that the only associative division algebras over the reals (permitting composition or not) are R,C,H of dimensions 1, 2, 4. In 1898 Hurwitz proved via group representations that the only quadratic forms permitting composition over the reals are the standard ones of dimension 1, 2, 4, 8; A.A. Albert later gave an algebra-theoretic proof over a general field of scalars (with an addition by Irving Kaplansky to include characteristic 2 and non-unital algebras). Only recently was it established that the only finite-dimensional real nonassociative division algebras have dimensions 1, 2, 4, 8; the algebras themselves were not classified, and the proof was topological rather than algebraic.

ONLY FOUR TYPES RHCO 1,2,4,8 ARE POSSIBLE

https://en.wikipedia.org/wiki/Hurwitz%27s_theorem_(composition_algebras)

In mathematics, Hurwitz's theorem is a theorem of Adolf Hurwitz (1859–1919), published posthumously in 1923, solving the Hurwitz problem for finite-dimensional unital real non-associative algebras endowed with a positive-definite quadratic form. The theorem states that if the quadratic form defines a homomorphism into the positive real numbers on the non-zero part of the algebra, then the algebra must be isomorphic to the real numbers, the complex numbers, the quaternions or the octonions. Such algebras, sometimes called Hurwitz algebras, are examples of composition algebras.

The theory of composition algebras has subsequently been generalized to arbitrary quadratic forms and arbitrary fields.[1] Hurwitz's theorem implies that multiplicative formulas for sums of squares can only occur in 1, 2, 4 and 8 dimensions, a result originally proved by Hurwitz in 1898. It is a special case of the Hurwitz problem, solved also in Radon (1922). Subsequent proofs of the restrictions on the dimension have been given by Eckmann (1943) using the representation theory of finite groups and by Lee (1948) and Chevalley (1954) using Clifford algebras. Hurwitz's theorem has been applied in algebraic topology to problems on vector fields on spheres and the homotopy groups of the classical groups[2] and in quantum mechanics to the classification of simple Jordan algebras.[3]

THE FOUR NORMED DIVISION ALGEBRAS- THE FOURTH THE OCTONIONS DIFFERENT

https://en.wikipedia.org/wiki/Octonion

In mathematics, the octonions are a normed division algebra over the real numbers, usually represented by the capital letter O, using boldface O or blackboard bold

\mathbb {O} . There are three lower-dimensional normed division algebras over the reals: the real numbers R themselves, the complex numbers C, and the quaternions H. The octonions have eight dimensions; twice the number of dimensions of the quaternions, of which they are an extension. They are noncommutative and nonassociative, but satisfy a weaker form of associativity, namely they are alternative.

Octonions are not as well known as the quaternions and complex numbers, which are much more widely studied and used. Despite this, they have some interesting properties and are related to a number of exceptional structures in mathematics, among them the exceptional Lie groups. Additionally, octonions have applications in fields such as string theory, special relativity, and quantum logic.

The octonions were discovered in 1843 by John T. Graves, inspired by his friend W. R. Hamilton's discovery of quaternions. Graves called his discovery octaves, and mentioned them in a letter to Hamilton dated 16 December 1843, but his first publication of his result in (Graves 1845) was slightly later than Arthur Cayley's article on them. The octonions were discovered independently by Cayley[1] and are sometimes referred to as Cayley numbers or the Cayley algebra. Hamilton described the early history of Graves' discovery.[2]

As shown by Hurwitz, the only normed division algebras over the reals are R, C, H, and O. These four algebras also form the only alternative, finite-dimensional division algebras over the reals (up to isomorphism).

SEDONIANS 16 DIMENSIONS- 16 SQUARES QUADRANT MODEL

https://en.wikipedia.org/wiki/Sedenion

In abstract algebra, the sedenions form a 16-dimensional noncommutative and nonassociative algebra over the reals obtained by applying the Cayley–Dickson construction to the octonions. Unlike the octonions, the sedenions are not an alternative algebra. The set of sedenions is denoted by

\mathbb {S} .

The term sedenion is also used for other 16-dimensional algebraic structures, such as a tensor product of two copies of the biquaternions, or the algebra of 4 by 4 matrices over the reals, or that studied by Smith (1995).

Like octonions, multiplication of sedenions is neither commutative nor associative. But in contrast to the octonions, the sedenions do not even have the property of being alternative. They do, however, have the property of power associativity, which can be stated as that, for any element x of

\mathbb {S} , the power

x^{n} is well defined. They are also flexible.

Every sedenion is a linear combination of the unit sedenions

e_{0},

e_{1},

e_{2},

e_{3}, ...,

{\displaystyle e_{15}}, which form a basis of the vector space of sedenions. Every sedenion can be represented in the form

…

x=x_{0}e_{0}+x_{1}e_{1}+x_{2}e_{2}+\ldots +x_{{14}}e_{{14}}+x_{{15}}e_{{15}},\,.

Addition and subtraction are defined by the addition and subtraction of corresponding coefficients and multiplication is distributive over addition.

Like other algebras based on the Cayley–Dickson construction, the sedenions contain the algebra they were constructed from. So, they contain the octonions (

e_{0} to

{\displaystyle e_{7}} in the table below), and therefore also the quaternions (

e_{0} to

e_{3}), complex numbers (

e_{0} and

e_{1}) and reals (

e_{0}).

The sedenions have a multiplicative identity element

e_{0} and multiplicative inverses but they are not a division algebra because they have zero divisors. This means that two non-zero sedenions can be multiplied to obtain zero: an example is (

e_{3} +

{\displaystyle e_{10}})(

{\displaystyle e_{6}} −

{\displaystyle e_{15}}). All hypercomplex number systems after sedenions that are based on the Cayley–Dickson construction contain zero divisors.

In algebra, Pfister's sixteen-square identity is a non-bilinear identity of form

https://en.wikipedia.org/wiki/Pfister%27s_sixteen-square_identity

(

⋯

)

(

⋯

)

⋯

{\displaystyle (x_{1}^{2}+x_{2}^{2}+x_{3}^{2}+\cdots +x_{16}^{2})\,(y_{1}^{2}+y_{2}^{2}+y_{3}^{2}+\cdots +y_{16}^{2})=z_{1}^{2}+z_{2}^{2}+z_{3}^{2}+\cdots +z_{16}^{2}}

It was first proven to exist by H. Zassenhaus and W. Eichhorn in the 1960s,[1] and independently by Pfister[2] around the same time. There are several versions, a concise one of which is

If all

x_{i} and

y_{i} with

i>8 are set equal to zero, then it reduces to Degen's eight-square identity (in blue). The

u_{i} are

The identity shows that, in general, the product of two sums of sixteen squares is the sum of sixteen rational squares. Incidentally, the

No sixteen-square identity exists involving only bilinear functions since Hurwitz's theorem states an identity of the form

with the

z_{i} bilinear functions of the

x_{i} and

y_{i} is possible only for n ∈ {1, 2, 4, 8} . However, the more general Pfister's theorem (1965) shows that if the

z_{i} are rational functions of one set of variables, hence has a denominator, then it is possible for all

n=2^{m}.[3] There are also non-bilinear versions of Euler's four-square and Degen's eight-square identities.

In mathematics, Euler's four-square identity says that the product of two numbers, each of which is a sum of four squares, is itself a sum of four squares.

For any pair of quadruples from a commutative ring, the following expressions are equal:

https://en.wikipedia.org/wiki/Euler%27s_four-square_identity

Euler wrote about this identity in a letter dated May 4, 1748 to Goldbach[1][2] (but he used a different sign convention from the above). It can be proven with elementary algebra.

The identity was used by Lagrange to prove his four square theorem. More specifically, it implies that it is sufficient to prove the theorem for prime numbers, after which the more general theorem follows. The sign convention used above corresponds to the signs obtained by multiplying two quaternions. Other sign conventions can be obtained by changing any ak to −ak, and/or any bk to −bk.

If the ak and bk are real numbers, the identity expresses the fact that the absolute value of the product of two quaternions is equal to the product of their absolute values, in the same way that the Brahmagupta–Fibonacci two-square identity does for complex numbers. This property is the definitive feature of composition algebras.

Hurwitz's theorem states that an identity of form,

(a12+a22+a32+...+an2)(b12+b22+b32+...+bn2)=c12+c22+c32+...+cn2

where the

THERE ARE FOUR FORMS OF THE IDENTITY- FOUR VARIABLES

https://en.wikipedia.org/wiki/Brahmagupta–Fibonacci_identity

In algebra, the Brahmagupta–Fibonacci identity[1][2] says that the product of two sums each of two squares is itself a sum of two squares

The identity is also known as the Diophantus identity,[3][4] as it was first proved by Diophantus of Alexandria. It is a special case of Euler's four-square identity, and also of Lagrange's identity.

This shows that, for any fixed A, the set of all numbers of the form x2 + A y2 is closed under multiplication.

The identity holds in the ring of integers, the ring of rational numbers and, more generally, any commutative ring. All four forms of the identity can be verified by expanding each side of the equation. Also, (2) can be obtained from (1), or (1) from (2), by changing b to −b, and likewise with (3) and (4).

Analogous identities are Euler's four-square related to quaternions, and Degen's eight-square derived from the octonions which has connections to Bott periodicity. There is also Pfister's sixteen-square identity, though it is no longer bilinear.

If a, b, c, and d are real numbers, the Brahmagupta–Fibonacci identity is equivalent to the multiplicativity property for absolute values of complex numbers:

⋅

(

)

(

)

{\displaystyle |a+bi|\cdot |c+di|=|(a+bi)(c+di)|.}

This can be seen as follows: expanding the right side and squaring both sides, the multiplication property is equivalent to

⋅

(

−

)

(

)

{\displaystyle |a+bi|^{2}\cdot |c+di|^{2}=|(ac-bd)+i(ad+bc)|^{2},}

and by the definition of absolute value this is in turn equivalent to

(

)

⋅

(

)

(

−

)

(

)

{\displaystyle (a^{2}+b^{2})\cdot (c^{2}+d^{2})=(ac-bd)^{2}+(ad+bc)^{2}.}

An equivalent calculation in the case that the variables a, b, c, and d are rational numbers shows the identity may be interpreted as the statement that the norm in the field Q(i) is multiplicative: the norm is given by

(

)

{\displaystyle N(a+bi)=a^{2}+b^{2},}

and the multiplicativity calculation is the same as the preceding one.

GOES UP TO 16 SQUARES- THERE ARE 16 SQUARES IN THE QUADRANT MODEL- REDUCES TO EULERS FOUR SQUARE QUADRANT

https://en.wikipedia.org/wiki/Degen%27s_eight-square_identity

In mathematics, Degen's eight-square identity establishes that the product of two numbers, each of which is a sum of eight squares, is itself the sum of eight squares. Namely:

First discovered by Carl Ferdinand Degen around 1818, the identity was independently rediscovered by John Thomas Graves (1843) and Arthur Cayley (1845). The latter two derived it while working on an extension of quaternions called octonions. In algebraic terms the identity means that the norm of product of two octonions equals the product of their norms:

∥

∥\|ab\|=\|a\|\|b\|. Similar statements are true for quaternions (Euler's four-square identity), complex numbers (the Brahmagupta–Fibonacci two-square identity) and real numbers. In 1898 Adolf Hurwitz proved that there is no similar bilinear identity for 16 squares (sedenions) or any other number of squares except for 1,2,4, and 8. However, in the 1960s, H. Zassenhaus, W. Eichhorn, and A. Pfister (independently) showed there can be a non-bilinear identity for 16 squares.

Note that each quadrant reduces to a version of Euler's four-square identity:

https://answers.yahoo.com/question/index?qid=20080430113538AAjEiQj

XM is a marketing term used to promote the product in relation to the two most popular modulation schemes in broadcast radio today, AM (amplitude modulation) and FM (frequency modulation). XM does not possess any technical meaning whatsoever.

CRUCIFIXION IN PLANET OF THE APES

http://www.startribune.com/war-for-the-planet-of-the-apes-is-a-total-victory/434303613/

Reeves gives the film the gravity of a historical war epic. In a vast military fortress, the Colonel looks down upon the world from a balcony supporting a huge American flag. With ramrod authority, he commands a legion of armed, devout followers toward a final solution to end the rise of the apes. His towering fortress wall is being built by starving ape captives brutally whipped to keep them in line. The Colonel gradually reveals his messianic religious motivations for the coming genocide, and also for one of the film’s queasiest and most horrible images, the numerous apes literally crucified at his outpost.

http://tvtropes.org/pmwiki/pmwiki.php/Film/WarForThePlanetOfTheApes

The x-shaped crucifixes in the Alpha-Omega camp look similar to House Bolton's. The fact that the camp is in a snowy field helps.

Maurice's promise to the dying Caesar is near identical to the promise made by Varinia to the crucified Spartacus.

CRUCIFIXION IN PLANET OF THE APES

http://tvtropes.org/pmwiki/pmwiki.php/Film/WarForThePlanetOfTheApes

Crucified Hero Shot: Alpha-Omega bound apes in x-shaped crosses, leaving them to die from hunger and exposure. Caesar himself suffers from this in the middle of the film, and the reason he was captured is because he tried to rescue his commander, Spear, himself crucified.

CAESAR CRUCIFIED

http://tvtropes.org/pmwiki/pmwiki.php/Film/WarForThePlanetOfTheApes

Messianic Archetype: Caesar, though he is closer to Moses than to Jesus, leading his “people” out of captivity and dying once they reach The Promised Land. Though Caesar is crucified at one point.

CRUCIFIED APES

http://sequart.org/magazine/43347/beneath-the-planet-of-the-apes-is-completely-nuts/

Beneath the Planet of the Apes is Completely Nuts

Turns out a bunch of humans are still alive, and they’ve remained relatively unchanged in this time. Except for their psychic powers of course. They also worship an unexploded atomic bomb. They separate Brent (who they were controlling earlier when he tried to murder Nova) from Nova and interrogate him. Meanwhile the apes march towards the forbidden zone. The apes are faced with a psychic illusion designed to deter them – a wall of flaming, crucified apes in front of a giant statue of their god. The statue of their god is, naturally, bleeding. Isn’t this franchise meant to appeal to a younger audience? Anyway they get past the illusion. The psychics, who, it turns out, are just wearing people masks and are actually deformed mutants, put Brent in a cage with Taylor and force them to fight to the death. It’s probably the best fight scene in the film, despite looking like Charlton Heston is fighting a diminutive clone.

CRUCIFIED FIGURE PLANET OF THE APES

http://www.uncleodiescollectibles.com/img_lib/01%20Planet%20of%20the%20Apes%20Crucified%20Gorilla%2001%203-27-5.jpg

http://www.uncleodiescollectibles.com/html_lib/planet%20of%20the%20apes/00036.html

Crucified gorilla figure from Beneath the Planet of the Apes. (TCF, 1970) This crucified gorilla figure, famously featured in a mutant-induced vision in Beneath the Planet of the Apes, is the only existing life-sized ape figure ever made for the original classic movie series.

http://www.nachtkabarett.com/SmashingPumpkins/Machina/UnusedArtwork

In this depiction, Hermes/Mercury also bears the globus cruciger in his left hand. This, like the caduceus and the winged attire, was commonly associated with him in many depictions. One such, below:

Hermes in the ocean, under the sun and moon, holding a herald's rod in his right hand and a globus cruciger in his left. From The Hermetic Museum

The globus cruciger (meaning, "cross-bearing orb") is exactly that: an orb topped with a cross. Wielded by Hermes and coupled with the power of his staff, it represents his omniscient and omnipresent power.

At the bottom of the SP image lays a pair of caduceus wings, quite likely inspired by an illustration by D. Stolcius von Stolcenberg, from 1624:

THEOSOPHICAL SOCIETY CROSS AUM- ANKH IS CROSS AND AUM IS THREE PLUS ONE- STAR OF DAVID DOUBLE TETRAHEDRON MERKABA

https://en.wikipedia.org/wiki/Theosophical_Society

https://en.wikipedia.org/wiki/File:Theosophicalseal.svg

The Society's seal incorporated the Swastika, Star of David, Ankh, Aum and Ouroboros symbols.

FOURTH TEMPLE

https://en.wikipedia.org/wiki/Phoenicia_under_Roman_rule

Economic prosperity led to a revival in construction and urban development; temples and palaces were built throughout the country, as well as paved roads that linked the main cities like Baalbeck and Berytus. Indeed, starting in the last quarter of the 1st century BCE (reign of Augustus) and over a period of two centuries (reign of Philip the Arab), the Romans built a huge temple complex in Baalbek on a pre-existing tell dating to the PPNB,[1] consisting of three temples: Jupiter, Bacchus and Venus. On a nearby hill, they built a fourth temple dedicated to Mercury.

ADDED FOURTH TEMPLE

https://en.wikipedia.org/wiki/Baalbek

By that time, the complex housed three temples on Tell Baalbek: one to Jupiter Heliopolitanus (Baʿal), one to Venus Heliopolitana (Ashtart), and a third to Bacchus. On a nearby hill, a fourth temple was dedicated to the third figure of the Heliopolitan Triad, Mercury (Adon or Seimios[62]).

THE THREE STONES- BUT THERE IS A FOURTH TRANSCENDENT STONE

https://en.wikipedia.org/wiki/Baalbek

The Tell Baalbek temple complex, fortified as the town's citadel during the Middle Ages,[91] was constructed from local stone, mostly white granite and a rough white marble.

The complex is located on an immense[vague] raised plaza erected 5 m (16 ft) over an earlier T-shaped base consisting of a podium, staircase, and foundation walls.[j] These walls were built from about 24 monoliths, at their lowest level weighing approximately 300 tonnes (330 tons) each. The tallest retaining wall, on the west, has a second course of monoliths containing the famous "Three Stones" (Greek: Τρίλιθον, Trílithon):[37] a row of three stones, each over 19 m (62 ft) long, 4.3 m (14 ft) high, and 3.6 m (12 ft) broad, cut from limestone. They weigh approximately 800 tonnes (880 tons) each.[140] A fourth, still larger stone is called the Stone of the Pregnant Woman: it lies unused in a nearby quarry 800 m (2,600 ft) from the town.[141] Its weight, often exaggerated, is estimated at 1,000 tonnes (1,100 tons).[142] A fifth, still larger stone weighing approximately 1,200 tonnes (1,300 tons)[143] lies in the same quarry. This quarry was slightly higher than the temple complex,[121][144] so no lifting was required to move the stones.

The pattern of the quadrant model is manifest in the naming, ordering, and structuring of the story of the sons of Jacob.
In the biblical story of the prophet, Elijah, the prophet runs to the mountain top where his experience is expressed in the quadrant model pattern. The account is of four incidents,

the fourth being a transcendent encounter with the voice of God.
*Square one: "A great and powerful wind tore the mountains apart and shattered the rocks before the Lord, but the Lord was not in the wind." The first square is always the most ephemeral and the least solid.
*Square two: "After the wind there was an earthquake, but the Lord was not in the earthquake". The second always builds on the first. The second is not yet the third. The third is always the most related to doing.
*Square three: "After the earthquake came a fire, but the Lord was not in the fire." The fire is related to doing. Fires seem solid, and produce action. Fire builds upon the first two.
*Square four: "And after the fire came a gentle whisper". The fourth is different from the previous three, and builds upon them. The fourth is the most related to God. Elijah recognizes the voice of God in this gentle whisper. The fourth never seems to belong.

The biblical book of Job fits the quadrant model pattern. Job has four comforters who come to help him in his distress following his loss of everything. The comforters are
*Square one: Eliphaz
*Square two: Bildad
*Square three: Zophar
*Square four: Elihu. Elihu is qualitatively different from the previous three. The first three persistently try to convince Job that his punishment is just, but Job will not listen. Elihu comes in once the first three have given up, elaborating on what the first three have said, but goes beyond them. The fourth is always different from the previous three, yet engulfs them. Finally the transcendent God enters to finish the story.
The biblical story of the three men in the “fiery furnace” expresses the quadrant model pattern. Shadrach, Meshach and Abednego are warned that if they do not worship the idol they will be thrown into a fire. In spite of being thrown into the fire they survive. Babylonian guards looking in see a fourth figure that they say looks like "a son of the Gods."
In the biblical book of Daniel is an account of a dream that fits the quadrant model pattern. In the dream Daniel sees four winds, and then four beasts.
*Square 1: "The first was like a lion, and it had the wings of an eagle. I watched until its wings were torn off and it was lifted from the ground so that it stood on two feet like a human being, and the mind of a human was given to it." The first square is related to the mind. This beast, an empire, is described as being like a lion. The shutting of the lion's mouth represents the destruction of the empire and the neutralization of the enemy of the Israelites. This lion is described as having a mind of a human. In Wilbur's model the first square is mind. The first square is the light. Idealists are very mental. Seventh day adventists think this beast is the Babylonian Empire.
*Square two: “And there before me was a second beast, which looked like a bear. It was raised up on one of its sides, and had three ribs in its mouth.

between its teeth. It was told, ‘Get up and eat your fill of flesh!’” The second square is the culture square; eating is a cultural, social activity that people do together. The second square is the word. The second square is social. People often eat with family and friends. Without social interaction people go crazy. Astronauts need social interaction; if they cannot have them they at least need some sort of life to connect with--like a plant. Guardians are very into belonging and culture. Seventh day adventists think this beast is the Persian Empire.
Square three: “After that, I looked, and there before me was another beast, one that looked like a leopard. And on its back were four wings like those of a bird. This beast had four heads, and it was given authority to rule.” The third square is the doer square--the body. The leopard is the doer, with authority to rule. The Quadrant 3 personality, the Artisan, likes authority and respect. Seventh Day adventists think this beast is the Greek Empire.
*Square four: "After that, in my vision at night I looked, and there before me was a fourth beast—terrifying and frightening and very powerful. It had large iron teeth; it crushed and devoured its victims and trampled underfoot whatever was left. It was different from all the former beasts, and it had ten horns." The fourth beast is different from the previous three, which is characteristic the fourth square. The fourth best is described as terrifying and powerful. The emotion associated with the fourth quadrant is fear, which helps to facilitate flow. Knowledge is power. The beast has iron teeth, which parallels Daniel’s other dream where the fourth part of the statue is the iron legs. This beast is different from the other beasts, having 10 horns, almost a transcendent quality. The fourth always transcends the previous three, which are always more similar. This pattern is also reflected in thinking, emotion, doing, and dreaming. Thinking and emotion and doing are very connected, while dreaming seems separate, but encompasses them. In revelations, in the new testament, the beast with the ten horns is described to be like a lion, bear, and leopard. In other words it encompasses the previous three beasts. The fourth is always different, but encompasses the previous three. In other words, the quadrant model code is packed into the bible. Seventh Day adventists think this beast is the Roman Empire, which contains elements of the Babylonian, Persian, and Greek Empires.

Daniel has another dream where he describes a statue. The statue is from King Nebuchadnezzar's dream. The statue has a
square 1: Head of gold. Daniel says that the head represents a kingdom. This kingdom is Babylon according to scholars. Babylon worshipped a god named Tammuz. Tammuz was symbolized by a cross. He supposedly died and resurrected. Scholars think that he was a precursor to Jesus, and that the stories of Jesus borrowed from the stories of Tammuz that predated him.
square 2: A body of silver. Daniel says that the body represents a kingdom. The kingdom is the Persian empire. After being ruled over by the Babylonian Empire Israel was ruled over by the Persian Empire. Persians had gardens called paradizas. These gardens were shaped as quadrants. Scholars think that the story of the garden of Eden was written during the time when Israelites were under the Persian Empire’s rule, and that the garden paradise was borrowed from the Persians paradizas. Persians also practiced sun worship.
Square 3: Legs of bronze. Daniel proclaims that the legs represent a kingdom. Many scholars think that this kingdom is the Greek Kingdom. Bronze is very strong. The third square is always strong and solid. The greeks were known for philosophy and worshipping 12 gods.
Square 4: Feet of iron and clay. Daniel says that the feet are a final kingdom that he says is different from the rest. The fourth is always different from the previous three. Scholars think this kingdom is the Roman Empire. Many scholars and churches like the seventh day adventists think that the antichrist and beast of the Bible is the Roman Catholic Church. The protestants believed this. The seventh day adventists argue this because they say that this Church adopted the cross as its main symbol like the Babylonians did. It is also argued that the Catholic Church changed worship to Sunday as a symbol of sun worship like the Persian Empire did. It is further argued that the Catholic Church adopted Greek philosophy. So the seventh day adventists say that the Catholic Church is an amalgamation of the Empires that Israel was ruled over by, and thus it is an enemy of Israel. It is argued that the book of Revelations, written by John, was a metaphorical polemic against Rome. I can make a good argument that the gospels themselves were an allegorical polemic against the Roman Empire. But that is hopefully for another book. It is interesting that it is argued that these Empires are beasts and enemies of Israel. But scholars also argue that the stories of the bible borrowed deeply from the pagan religions and cultures of these empires. For instance, it is argued that the Israelites borrowed stories and material from pagan cultures around them and placed them into their own stories. For instance, the flood story of Noah is said to have been borrowed from the Sumerians as well as the story of Moses being put in the river. Also scholars argue the prophet Daniel himself was a character borrowed from pagan sources. I think that it can be safely argued that the Bible has similar content in its mythologies to the pagan Empires that ruled over the Israelites and surrounded the Israelites, and the Bible is also antithetical to these pagan Empires and mythologies, and tries to represent itself as contrary to them. So it borrows material from pagan mythologies and simultaneously undermines the pagan mythologies. Jung would argue similarities in mythologies is due to a universal unconscious and shared archetypes that all of humanity has in common.
The stories of Amos in the Bible fit the quadrant model pattern. There are twelve minor prophets and four major prophets in the BIble. The first twelve correspond to the first three quadrants that are very connected. This is like the twelve fermions of the standard model of particle physics. The four major prophets fit into Quadrant 4. They are different from the previous twelve, yet they encompass them. This is like the Bosons in the standard model of particle physics. Amos constantly repeats the principal behind the quadrant model pattern-- there are three that are very similar, but a fourth that is different, yet encompasses them. God says to Amos,
'“For three sins of Damascus,
even for four, I will not relent.
Because she threshed Gilead
with sledges having iron teeth,
4 I will send fire on the house of Hazael
that will consume the fortresses of Ben-Hadad.
5 I will break down the gate of Damascus;
I will destroy the king who is in[b] the Valley of Aven[c]
and the one who holds the scepter in Beth Eden.
The people of Aram will go into exile to Kir,”

Amos says that God is saying this; he is speaking through God, saying “for three even for four...”
Amos then continues,
"'For three sins of Gaza,
even for four, I will not relent.
Because she took captive whole communities
and sold them to Edom,
7 I will send fire on the walls of Gaza
that will consume her fortresses.
8 I will destroy the king[dom] of Ashdod
and the one who holds the scepter in Ashkelon.
I will turn my hand against Ekron,
till the last of the Philistines are dead,”
says the Sovereign Lord.'
Again Amos is saying, “for three sins of Gaza even for four”. This is subtly representing the nature of the quadrant. The three are for certain; the fourth is different and sort of questionable. But the fourth exists. He says, "even for four". This pattern of speaking of “three, even four” is repeated several more times. This statement subtly describes the quadrant model pattern where the three are connected and certain, and the fourth is different, described as, "even four".
The prophet Zechariah in the Bible has a vision in which he sees four horns that scattered Israel, Jerusalem, and Judah. These four horns represent the quadrant. But then Zechariah describes four craftsmen that scare away and destroy these four horns that are going to destroy Israel and Judah and Jerusalem. This harkens back to the dreams of the four kingdoms. There are four craftsmen that stop the destruction of Israel. These four craftsmen are like the prophets who stop the destruction of Israel by returning Israelites to the law of God, also destroying by converting the empires that are against Israel. Zechariah also has a vision of two lamp stands. God says that these are the two who are anointed to serve the Earth. The two lamp stands are said to represent Moses and Elijah. Moses represents the written law, and Elijah represents the spiritual law. Jesus is on top of a mountain when Moses and Elijah, along with God appear to him. This fits the quadrant model in that these are four great figures. Jesus in the New Testament is not represented as different from the Old Testament but as doing the same thing that Elijah and Moses did.
Later Zechariah has a vision where he sees four chariots. These four chariots fit the quadrant model pattern. Zechariah goes, "I looked up again, and there before me were four chariots coming out from between two mountains—mountains of bronze. 2 The first chariot had red horses, the second black, 3 the third white, and the fourth dappled—all of them powerful. 4 I asked the angel who was speaking to me, “What are these, my lord?”'
This vision fits the quadrant model pattern.
*Square one: Red horses
*Square two: Black horses
*Square three: White horses
*Square four: Dappled horses.

The first three are solid and similar colors; the fourth is different from the previous three, yet it encompasses them. The fourth is dappled, meaning that it is red, black, and white. The fourth is always separate, yet encompasses the previous three, containing the same elements.
The New Testament portion of the Bible is divided into four quadrants.
*Square one: The Gospels--about the life of Jesus.
*Square two: Origins of the Christian Church. The second square is always about a family and most related to family--it is culture.
*Square three: Epistles--letters written by Paul and the apostles of Jesus, telling people what to believe and how to live. The third square is doing.
*Square four: Revelation—metaphorical and philosophical. The fourth never seems to belong with the other three. It can be viewed as an allegory of the Roman Empire trying to destroy the Church, with the Word of God and prophets fighting against the beast.

During the time of the Roman Empire there were four major sects of Judaism that fit the quadrant model pattern.
*Square one: Sadducees—composed primarily of upper aristocracy Jews, many of whom served as priests in the temple and worked with the Roman authorities. They stressed the temple and were very involved with the idea of sacrifices to God.
*Square two: Pharisees--followed the Torah and oral torah, stressing conformity to the law. The second square is faith and family, and is the most concerned with conformity to the law.
*Square three: Essenes--thought the rest of Israel had become impure due to sin; they often left the rest of Israel to form their own monastic communities. The third square is the individual and the doer. They were very apocalyptic in their views, believing that the world would come to an abrupt end, and most of the world, including most of the Jews who had gone astray, would be destroyed. The third square is considered bad and destructive.
*Square four: Fourth Philosophy--believed that Israel should only be for Jews and Israelites. The fourth philosophy is different from the previous three, a common characteristic of the fourth square in the quadrant model.

The four gospels fit the quadrant model pattern. The first three synoptic gospels are Mark, Matthew, and Luke. The fourth has been called the "maverick" gospel because it is very different from the other three--the fourth square in the quadrant model is always different from the first three. The synoptic gospels are extremely similar; that is the nature of the first three squares. They are extremely interconnected, often sharing completely whole passages. But Matthew emphasizes Jesus's Jewishness. trying to make Jesus fit in as the Jewish Messiah. Historians think that there are four sources out of which the gospels emerged. They are called Mark, Q, M, and L.
*Square one: Mark—the earliest account--about Jesus, the suffering son of God who is abandoned, distraught, and forsaken. The first square does not yet belong. This is the nature of the first square. In Luke Jesus is depicted as calm, collected, and in control. This is more the nature of the third square. Artisans

who are the third square are more sure of themselves than Idealists who are the first square. In Mark Jesus's family thinks that Jesus has gone crazy and they are worried about him. Jesus says that his family is those who do the will of His Father. The leaders of the Jews think that Jesus is possessed by a devil. Really, we know that the Pharisees are against Jesus because he is bringing people back to God, and many pharisees at the time had assimilated into Rome and feared teaching the torah and hid the Truth because it would reveal that they are liars and hypocrites and really just selfish greedy pawns of the Roman Empire system. Jesus's own disciples it is said, do not know who he is and what his deal is about.
*Square two: Matthew--labels Jesus as the Jewish Messiah. The second square is more about conformity, belonging, and family. The emphasis of Matthew is that Jesus is a Jew and fits the criteria to be the Messiah, with a genealogy that depicts him as a son of Abraham, which emphasizes Jesus's Jewish lineage.
*Square three: Luke—the third to be written--depicts Jesus as the savior of the entire world. The third square is the doing square. Saving the world is an action that Jesus is doing throughout this gospel. This square builds upon the last square--in this gospel Jesus is not just for the Jews, but is depicted as being a savior for the whole world. In Luke Jesus has more authority. That is the nature of the third square. The Artisan personality type, which is the third square, gets respect and likes authority. Jesus in this gospel is worshipped even as an infant, by the three wise men. Luke's genealogy goes all the way back to Adam and Eve. The third square connotes being destructive or bad, but it is also about respect; Jesus is depicted as sure of himself. This is more the nature of the third quadrant. Some say that Luke also emphasizes more the poor and needy and women, taking a broader scope than the other two gospels.
*Square four: John--John emphasizes Jesus as the man from heaven. The fourth is always different from the previous three and has a transcendent quality to it. The fourth connects to the fifth, which is directly associated with God. The fourth is transcendent and has a transcendent quality to it. The fourth is always different; it is very philosophical and theological, with a mysterious quality to it, a typical fourth square characteristic. It contrasts greatly with Matthew. This makes sense in terms of the quadrant model; the second and fourth squares are dynamic opposites. In Matthew Jesus refuses to perform signs unless it is to help people. The second square is belonging, and it is to help people. In John Jesus performs signs to prove his identity as the messiah. Historians think that this gospel was written at a time when Christianity was moving away from the Jewish people, becoming more involved with gentiles, and that it is therefore starting to emphasize Jesus as divine and apart from just a Jewish messiah.

In the gospel parable of the sower the quadrant pattern is very clear. Jesus is reported to have told a story of seeds being planted in four separate places, yielding four different results.
*Square one: Seed falls on the path, and the birds eat it. The seed represents

the Word of God. This fits the Idealist type, who sense and perceive--they do not have a firm grasp of things, so the word is stolen and not completely understood..
*Square two: Seed falls along the rocky soil. The plants spring up quickly, but the soil is shallow allowing the sun to scorch and destroy them. This is the nature of the Guardian, who has a firm grasp on things, but no roots. Beliefs and faith are more firm, but are not rooted, and can easily be false.
*Square three: Seed falls among the thorns, and are choked and killed. The third square is the bad, destructive square. The third is the most violent. Artisans are associated more with being bad and violent.
*Square four: Seed fell on good soil, where it produced a crop—a hundred, sixty, or thirty times what was sown. The fourth is always different from the previous three. This seed is in good soil, and it multiplies. The nature of this soil is a lot different from the previous three and transcends them. This is the nature of the fourth square.

Hinduism has characteristics of the third square as it encompasses the previous three world religions, stating that the prophets of these religions are avatars or holy men, and that all gods are expressions of the concept of Brahma, which means “Being” (or Krishna) (a characteristic of the fourth square of Quadrant 5). Brahma has four arms and four heads reminiscing the quadrantfrom which it is thought

the four vedas emerged--the four vedas, written in sanskrit, are the foundational texts of Hinduism. These texts fit the quadrant model pattern.
*Square one: the Rigveda--hymns recited by the priest. The first square is the mind, and is related to the priest.
*Square two: Yajurveda--formulas to be recited by the officiating priest. The second square is about order and homeostasis--formulas are concerned with order and homeostasis. The second square is culture.
*Square three: Samaveda--formulas that are to be sung by the chanting priest. The third square is doing. The third square is action.
*Square four: Atharaveda--contains spells, incantations, charms, and speculative hymns. The fourth square is contemplation, and transcends the others. Contemplation is about speculation.

According to Hindu theology there are four ages. These are
square 1- The Satya Yuga. During this era virtue reigns and there is not sin. The first square is always good. Also this era is marked by wisdom which is a quality of the first square. The first square is mental.
square 2- Treta Yuga. During this era there is three quarters virtue and one quarter sin. The second square is always good.
square 3- Dvapara Yuga. During this era there is one half sin and one half virtue. The third square is always bad
square 4- Kali Yuga. During this era there is one quarter virtue and three quarters sin. Hindus say that this is the current era. The fourth square is death. The fourth square is the worst.
Each age is further divided into four sections each making a total of 16 squares, which is the quadrant model.

There are four Mayan codices. Again the fourth is always different from the previous three. The fourth codex is questionable. They are
square 1- The Madrid codex
square 2- The Dresden codex
square 3: The Paris codex
Square 4: the Colier codex

There are examples of myths in other cultures that express the quadrant model. According to Hesiod's theology there are four primordial Greek deities.
*Square one: Chaos. This is the void.
*Square two: Gaia. This is mother Earth. Gaia is homeostasis and order. That is the second square.
*Square three: Tarturus. This is the underworld. The third square is bad.
*Square four: Eros. Procreation. The fourth square is knowledge/sex.

The Greek poet Hesiod describes ages of men that fit the quadrant model pattern. They are
Square one: The golden age. Hesiod states that men of this age are wise. They do not have to work and they live to a very old age. The first square is characteristically related to wisdom. The first square is the mind. The first square is good and conservative.
Square two: The silver age. Hesiod describes that this age is an age where the people worship the gods but they are ultimately kicked out of this age due to impiety. The second square is related to religiosity.
Square three: The bronze age. Hesiod depicts men of this age as hard and tough and warlike. This age is characterized by war. The third square is the most physical and it is destructive.
Square four: The heroic age. Notice how the first three ages are named after metals. The fourth square is always different. During this age men live with demigods and heroes. The fourth square always has a sort of transcendent quality to it, that makes it different from the first three.
Square five: The iron age. This is the current age where Hesiod declares might makes right and the world is full of evil.

HISTORIANS SEE THE SEVEN KINGS AS A FOUR PLUS THREE- THEY THINK THE FIRST FOUR ARE DIFFERENT AND EVEN ARGUE THERE WAS ONLY FOUR KINGS

The seven Kings of Rome fit the quadrant model pattern. They are
Square 1: Romulus. Romulus was a good king. The first is always good.
Square 2: Numa Pompilius. He was a king who established order, structure and religion in Rome. The second square is order.
Square 3: Tullus Hostilius. His name means hostile. The third square is always bad and destructive. He was a warlike king.
Square 4: Ancus Marcius. He was a philosophical king. The fourth square is related to intelligence.
Square 5: Lucius Tarquinius Priscus. This is the first square of the second quadrant. Priscus was very into order and political structure, structuring things like the senate.
Square 6: Servius Tullius. He built temples and was very popular. Square 6 is the second square of the second quadrant. This square is the most associated with order and religiosity. He helped people like the poor. He served people, hence the name Servius. The sixth square is related to serving people. It is faith. The sixth personality type is the ESFJ who loves to help people.
Square 7: Lucius Tarquinius Superbus. He is the third square of the second quadrant. The third square is always bad. He was proud and the monarchy of Rome ended with him.

Plato's famous allegory of the cave was designed to describe reality. He said that apparent reality is only a shadow of true reality, which he called the World of Forms, an eternal and unchanging reality. Socrates said that reality derived from One Form. The theory proposed by the Quadrant Model of Reality is that this form is the form of the cross.
Plato used an illustration called the divided line to describe the nature of reality. The divided line fits the quadrant model pattern.
*Square one: This is the square of imagining. According to Plato this is the square of sensation and perception. The first quadrant is the sensation and perception quadrant. Plato describes that the world that is sensed and perceived is an illusion' this level of knowledge is therefore unsatisfactory. Plato said that sensations and perceptions are just shadows of the true reality.
*Square two: Plato labels this section of the divided line belief. The second quadrant is belief and faith. Plato said that beliefs are about physical things in the physical world. He says that this section is also faith, that beliefs are not grounded in reality, and that physical things are not real.
*Square three: Plato labels this section of the divided line thinking. The third quadrant of the quadrant model is the thinking and doing quadrant. Plato describes that people at this level of knowledge perform mathematical reasoning. Mathematics is a form of doing; when you perform mathematics, a part of the performance is pointing and gesturing and writing itself. A part of thinking is actions performed with the body. This is the doing square.
Square four: Plato labels this square knowledge. The fourth quadrant of the quadrant model is the knowledge quadrant. Plato says that knowledge is recognition of the world of Forms, and that reality is a reflection of a true reality, which is a world of Ideas and Forms. He says that knowledge of this reality comes through conversations with people, which he calls dialectic. The fourth square is social, and points beyond itself. Plato's dialogues themselves are his written records of conversations between Socrates and other people. Socrates would often take an opposite viewpoint of another person, and in his argument eventually try to come up with the Truth. This contrasted with sophists who would argue to try to prove a point that they were trying to make and didn't care about discovering the Truth. Plato says that ultimate knowledge is knowledge of the Form of the Good; out of this Form everything derives. This form is the form of the cross and the quadrant model pattern which permeates from it.

There is a free will vs. determinism debate in religion. According to Calvinist doctrine the Bible states that everything is predetermined by God, including whether or not one believes in God, which makes not believing in God undeserving of punishment. In the free will vs. determinism debate there are four possibilities.
*Square one: Hard determinism--everything is predetermined; there is no free will. This corresponds to the Idealist who is abstract and cooperative. Abstract people see patterns and make connections, recognizing that things are determined. They understand the quadrant model, and realize that it has determined everything. But they are cooperative, follow orders, and are very submissive to authority. Therefore they do not have free will. They worry more about doing what brings social harmony and maintains the status quo and inaction.
*Square two: Hard incompatibilism--things are not determined; there is no free will. This corresponds to the Guardian who is concrete and cooperative, and unable to see the connections and oneness of things. The tendency is to think that things are not determined. Guardians are also cooperative, focusing on behaving and belonging. Therefore they are more concerned with following rules and orders, therefore they do not have free will.
*Square three: Libertarianism--things are not determined; there is free will. This corresponds to the Artisans who are concrete and utilitarian, and unable to see connections. They do not see the oneness of things, and do not think things are determined. But they are utilitarian. They do what they want to do and what works. They are more spontaneous, not caring about the rule book or social harmony and maintaining the social order.
*Square 4: Compatibilism--things are determined; there is free will. This corresponds to the Rationals who are abstract and utilitarian. Abstract people recognize that things are connected; they recognize the quadrant model and see that everything is determined by this principal. There cannot be anything other than that which elucidates the quadrant model pattern. Only one thing can elucidate the quadrant model pattern. Therefore everything is determined.Rationals are also utilitarian. They therefore do not care about social harmony, following the rule book, and maintaining the status quo. They do what they want, and do what they think works and what does work. Therefore they have free will.

Joseph Smith, who founded Mormonism, carried what was called the Jupiter stone. The Jupiter stone contained sixteen squares.

There is a popular book called the four Christian cults, which describe four groups of Christianity that are described by some as cults, which are square 1 Christian science, square 2 Jehovahs’ witnesses, square 3 seventh day adventists and square 4 Mormons
Ancient alien hypothesizers theorize that the gods of ancient cultures were actually aliens. A famous abduction experience is the Allagash experience, where four people were abducted. Two of them were twins. The twins represent the duality.

I TOOK A CINEMA CLASS AT UCSD BY A FAMOUS CINEMA TEACHER AND HE SAID THAT CINEMA BORROWS FROM THE FOUR PRIMARY DOMAINS OF ART AND THAT HISOTRICAL PERIODS IN CINEMA ARE CHARACTERIZED BY ONE OF THE FOUR PRIMARY DOMAINS (ALTHOUGH HE THOUGHT MODERN CINEMA WAS JUST TRASH AND NOT ART AT ALL)

The four primary domains in the Arts are a manifestation of the quadrant model pattern of four.
*Square one: Painting. Square 1 is the light, and is the mind square. The sense associated with this square is vision. Vision is related with painting. The third quadrant is all about action. Painting is an action, a form of expression and some would argue a form of thought. One who draws out a geometric math problem is performing art. The act of painting is like the act of thinking, and a way to express emotions. Van Goh said he painted his dreams. The third quadrant is thinking, emotion, doing, and dreaming. Art is a way to express thoughts and emotions, and is even a form of thinking and emoting by the artist. The first square component of physics is space. Space is associated with painting--painting is color in space.
*Square two: Music. Square 2 is the word, the culture square. The sense associated with this square is hearing. Hearing is related with music. Music has been described as emotion expressed through sound. The definition of music is sound in time. The second square component of physics is time. Creating music is an action. People create music with instruments. The voice is an instrument. Sound is produced through the vibration of air molecules through sound waves. It is interesting that color is the product of light waves and photons, so painting and music are very similar--they are both the products of the vibrations of molecules, producing perceptual interpretations in the people that observe them. I also described how the third square is sexual in nature.
The third quadrant is the dreaming quadrant. Freud discussed that dreams are sublimations of sexual instincts and impulses in the subconscious. Thoughts, and emotions are shaped by sexual drives.
*Square three: Dance. The third square is the flesh square. The third square in Wilbur's model is the body square. The third square is the doing square The mode of art associated with the body and movement is dance. The quadrant model is a holistic model, so painting and music are forms of dance. To paint and make music requires body movement. Pollack, a famous painter, was known for making paintings where he splattered paint over the canvas. While he made these paintings he would listen to classical music by Bach, who was a German composer of the enlightenment. As he listened to this music he would dance.
*Square four: Literature. The first square is the light--that is painting. The second is the word--music. The third is the flesh--dance. The fourth is the true word--literature. The Bible and the Odyssey were originally oral, and were passed on through oral tradition. Socrates warned that writing would make memory worse. The fourth sense is taste, associated with the mouth, which is associated with speaking. Literature, originally oral, is different from the previous three modes of art, yet it encapsulates them; it is the fourth square mode of art--the nature of the fourth square. The fourth square is separate from the previous three, yet it encompasses them.

ANCIENT PHILOSOPHERS SUCH AS PROCLUS ARGUED THAT EUCLIDS FOURTH POSTULATE WAS DIFFERENT AND NOT A POSTULATE, ALTHOUGH IT HAS BEEN UPHELD TO BE A POSTULATE AND TRUE. THE FOURTH IS ALWAYS DIFFERENT. HOWEVER THE FIFTH HAS BEEN PROVEN TO BE FALSE. THE FIFTH IS ALWAYS QUESTIONABLE

ANCIENT PHILOSOPHERS LIKE PROCLUS QUESTIONENED THE FOURTH POSTUALTE SAYING IT IS NOT A POSTULATE AND ALTHOUGH MODERN PHILOSOPPHERS AGREE THE FOURTH IS DIFFERENT THEY UPHOLD IT AS TRUE AND A POSTULATE BUT THE FIFTH IS QUESTIONABLE AND ULTRA TRANSCENDENT AND HAS BEEN PROVEN TO BE FALSE ONLY BEING TRUE UNDER CERTAIN GEOMETRIES, like EUCLIDEAN- BUT IT HAS BEEN ARGUED THAT THE FIFTH GAVE BIRTH TO NEW GEOMETRIES AND MAYBE EUCLID PROPOSED IT INTENTIONALLY IN ORDER TO SPUR NEW GEOMETRIES

Mathematics is one attempt to look at and understand the physical world. Euclid, a famous Greek mathematician, is called the "Father of Geometry". His book, “The Elements” is one of the most popular books in the world, behind only the Bible. It is not only very philosophical but also the foundation for all geometry. And it fits the quadrant model pattern. The book contains the five postulates underlying all geometry. Placing them within the quadrant model pattern reveals the underlying Form of Existence.
*Square one: “A straight line can be drawn from one point to another.” This is directly connected with the next square.
*Square two: “A straight line can be extended continuously.” The first two squares are a duality; they are related to each other in that both are related to lines. The first two squares are not yet solid. The third square is the solid square.
*Square three: “A circle can be created with an equal radius from a center point.” The third square is always the solid square. The first two squares are about lines; the third square is about a circle, which is an enclosed shape, therefore it is the solid square.
*Square four: “All right angles are equal to each other.” What is interesting is that a right angle is the angle found in the four quadrants. The fourth square is related to the divine, and is transcendent. The fourth is always different from the previous three. The fourth is related to the quadrant itself.
*Square five: The fifth square is the parallel postulate. The idea behind this postulate is that if two straight lines are intersected, and the line that intersects them intersects at right angles, then the two lines are parallel and will never meet. The fifth is always questionable. It turns out that the fifth postulate is false. It is good that Euclid never really needs to use this postulate. Again, the fifth is always questionable. The fourth often seems not to belong, but the fifth does not belong at all. Also the fourth points to the fifth. The fourth is about right angles, and the fifth is about parallel lines that are produced when a line intersects them to form right angles.

Here's an excerpt from my book QMR

For thousands of years it was thought that the fifth postulate was correct. But around the time of Einstein this postulate was questioned. Two people came up with the same conclusion independently. Some question why Euclid made a fifth postulate if he did not really need it. Some have said that Euclid intentionally did this so people would later try to prove it wrong, and in so doing discover new geometries. Others say that Euclid’s fifth postulate is not wrong, but is wrong only if other spatial planes are added. They say on a Euclidean plane the fifth postulate is correct, so it is incorrect to say the fifth postulate is wrong. But the point is the fifth is always questionable. That is the nature of the quadrant model. The fourth is transcendent, and fifth is ultra-transcendent.
Euclid's elements are also founded upon five axioms that fulfill the quadrant model pattern. They are as follows:
*Square one: “Things that are equal to the same thing are equal to one another” (Transitive property of equality).
*Square two: “If equals are added to equals, then the wholes are equal” (Addition property of equality). The first two squares are a duality, and are also conservative. They are about maintaining and building.
*Square three: “If equals are subtracted from equals, then the remainders are equal” (Subtraction property of equality). The third square is about destruction--it is about subtracting.
*Square four: “Things that coincide with one another are equal to one another” (Reflexive Property). The fourth square seems different and seems like nothing is there.
*Square five: “The whole is greater than the part.” The fifth square is always questionable. In fractal mathematics it has been discovered that the whole is not greater than the part; the whole can be in the part. Also in quantum mechanics it has been discovered that particles can decay and create wholes that are greater than the parts. Again the fifth is questionable. But using the five postulates that fit the quadrant model pattern and the five axioms, Euclid derived all of geometry.

The whole is greater than the part
Euclid's book itself fits the quadrant model pattern; it is organized in terms of the quadrant model pattern.
Quadrant 1: The first four books of Euclid's Elements fit the qualities of the first four squares of the quadrant model.
*Square one: Book 1--discusses the five postulates and five axioms.
*Square two: Book 2--talks about geometric algebra and finding the square of a number.
*Square three: Book 3--talks about circles. The third square is when things start getting solid--the first two squares are not solid, but in the third square things are a little more solid. Circles are enclosed.
*Square four: Book 4--incircles and semicircles, along with regular polygons, are described. The figures are flat, more solid and complex. The fourth square is more abstract. Each square builds upon the last.
Quadrant 2: The second four books of Euclid's book fit the qualities of the next four squares of the quadrant model. These squares are:
*Square one: Book 5--discusses proportions and magnitudes. The second quadrant, about relationships, is the cultural quadrant, and deals with relationships. Proportions and magnitudes are about relationships between things. The second quadrant is about relationships.
*Square two: Book 6--applies proportions to geometry. Square 6 is the second square of the second quadrant. The second square is about structure and relationships. Here proportions are discussed, which are about relationships;

proportions are discussed in relationship to geometry, which is about shape and form. The second square is related to form, and its shape and structure.
*Square three: Book 7--deals with elementary number theory. It is more related to doing, but number theory is still about relationships between numbers. The second quadrant deals with relationships.
Square four: Book 8--deals with proportions in number theory and geometric sequences. The second quadrant again is about proportions, which is about relationships. But these proportions are related to number theory, and number theory is more related to doing.
Quadrant 3: Book 9, the third of four books of Euclid's Elements fits the qualities of the next four squares of the quadrant model.
*Square one: Book 9--talks about the infinitude of prime numbers, the sum of a geometric series, and the construction of even perfect numbers. The third quadrant is more wild. The first square of the third quadrant is thinking. Thinking is wild; it is also doing. Now Euclid is performing serious mathematics.
*Square two: Book 10--an attempt to classify irrational magnitudes by using the method of exhaustion. This is the second square of the third quadrant. The tenth square is the emotion square. The third quadrant is wild. The third quadrant is also doing. But we are not yet at the third square of the third quadrant. The third square of the third quadrant must be the most solid, because the third is the most solid and is the most related to doing. The third square of the third quadrant is doing.
*Square three: Book 11--finds the volume of parallelepipeds. Volume is the amount of area within a solid structure. Physical objects occupy space; the nature of the third square is the most solid.
*Square four: Book 12--studies the volume of square one: cones, square two: pyramids, square three: cylinders and square four: spheres. Book 12 is the fourth square of the third quadrant. It is therefore still solid, but it is even more abstract than the third square of the third quadrant, because the fourth square is always more abstract and complex.
Finally Quadrant 4: Book 13- is the first square of the fourth quadrant. Quadrant 4 is the contemplation square. Here Euclid discusses the Platonic solids. Euclid sees these as divine, as did Plato. These are the five platonic solids that fit the quadrant model pattern. The fourth quadrant is divine and transcends the previous three.

Aristotle is known as one of the founders of logic; he invented the logic square. Logic squares fit the form of the quadrant model of reality. In logic squares there are four proposition forms. They are:
*Square one: the A proposition, called the universal affirmative, and goes “Every S is P”. For instance every collie is a dog.
*Square two: The E proposition--called the universal negative. It is translated “No S are P.” For instance, no humans are dogs.
*Square three: The I proposition--called the particular affirmative. It is translated “Some S are P.” For instance, some four legged animals are dogs.
*Square four: O is the particular negative--translated, “Some S are not P.” For instance, some four legged animals are not dogs.

Aristotle postulates that there are four logical relationships among the propositions. These fit the quadrant model pattern. They are:
*Square one: Contraries. Universal statements are contraries. Contrary statements cannot both be true at the same time. For instance, it cannot be said that every collie is a dog and no collies are dogs. A contradictory is true when its opposite is false. For instance, if no collies are dogs is false, then collies are dogs must be true.
*Square two: Subcontraries. Particular statements are subcontraries. Subcontraries can both be true, but they cannot both be false. Contraries and subcontraries are a duality. The first two squares are always a duality. For instance it cannot be that “some ghosts are real is false” and “some ghosts are not real is false”. If one is false then the other must be true.
*Square three: Sublanterns--when the universal statement implies the particular statement. For instance, all men have penises is true. This implies that some men have penises.
*Square four: Contradictory. Universal affirmatives and particular negatives are contradictories. For instance, if every A is B, not some A is not B. An example of this is, every dog is an animal. Then it cannot be that some dogs are not animals; these are contradictories. It is interesting that the concept of contradictories has been questioned. The fourth square never seems to belong. Set theory has proposed a phenomena called, empty sets, where it is proposed that contradictories are possible. The fourth never seems to belong. These different propositions are often represented in pictorial forms by venn diagrams. Logic squares are the foundation of logic, and they fit the quadrant model pattern.

In mathematics and logic an extremely important concept is the concept of Truth Tables. Truth Tables take the quadrant model pattern. There are four possibilities in an atomic Truth Table.
*Square one: Proposition one is true, and proposition two is true.
*Square two: Proposition one is true, and proposition two is false.
*Square three: Proposition one is false, and proposition two is true.
*Square four. Proposition one is false, and proposition two is false.
There are five standard connectives used to form compound propositions from atomic propositions. These are:
*Square one: Disjunction--called inclusive OR statements. An example is, the player has to score 50 points per game or make five three pointers per game to be the MVP. The idea is if either the player scores 50 points per game, or makes five three pointers, then he will be the MVP. He does not need to do both; he must do only one. Proposition one is labeled P. Proposition two is labelled Q. If P is True and Q is true then the statement, R, is true. If P is True and Q is False then the statement R is true. If P is False and Q is true then the statement R is true. IF P is false and Q is false then the statement R is false.
*Square two: Conjunction--called AND statements. An example of a conjunction is if the player scores 50 points a game, P, and makes five three pointers per game, Q, then he will get the MVP. Again there are four possibilities. If P is true and Q is true, then R is true. If P is true and Q is false then R is false. If P is false and Q is true then R is false. If P is false and Q is false then R is false. Disjunctions, which are “or statements”, and conjunctions which are “and statements” are the duality.
*Square three: Conditionals--also called implications. These are if/then statements. For instance, if P, then Q. Another way to say it is, P implies Q. Examples would be, if the man scores 50 points it implies that the man made five three pointers. This is also called modus ponens. If P is true then Q must be true. It is impossible for Q to be false if P is true. If Q is false, P must be false. If P is false then Q can be true or false. P implies Q is always true if P is false. This is confusing, but these are the rules. The truth values are, if P is true

and Q is true then R is true. If P is true and Q is false then R is true. If P is false and Q is true then R is true. If P is false and R is false, Q is true.
*Square four: Biconditional. This is, if and only if, the fourth square. The fourth is always different. This is a statement of equivalence. If the truth values of P and Q are identical then the statement is true. If P is true and Q is true, R is true. If P is true and Q is false, R is false. If P is false and R is true then Q is false. If P is false and R is false then Q is true.
*Square five. Negation--a possible fifth square. It is very different from the previous four, and is questionable. It means “not”. There are two truth values for an atomic negation truth table. If P is true then not P is false. If P is false then not P is True.

The geometric nature of calculus also fits the quadrant model pattern. In statistics there are four options. These options fit the quadrant model pattern.
*Square one: You reject H not and H not is true. This is a type 1 error.
*Square two: You fail to reject H not and H not is true. This is no error.
*Square three: You reject H not and H not is false. This is no error.
*Square four: You fail to reject H not and H not is false. This is a type 2 error. This chart is the foundation of statistics, and the type of error that occurs determines statistical probabilities.

Empedocles was a famous Greek pre-Socratic philosopher. He said that everything was based around the conflict of love and strife. He proposed a four stage cosmic cycle around the principal of love and strife.
*Square one: Pure domain of love, harmony. During this stage Empedocles says there is no life. This is the ideal stage. The first square is the Idealist. Life is bad, and in this stage there is not yet life.
*Square two: Contention between love and strife. During this stage there is a presence of life. There is a contention, but there is not yet any outright conflict. The second square is homeostasis. There is order in this square, but things are about to change.
*Square three: Pure domain of strife--chaos. Empedocles says in this stage there is outright conflict. The third square is the action square, it is bad and destructive. It is the doing square. There is no life in this stage, according to Empedocles.
*Square four: Contention between strife and love. Life is present in this stage. This is different from stage two in that in it love is fighting strife, and in stage four strife is fighting love. The fourth square is pointing beyond life to death.

The quadrant model points to Parmenides's philosophy--things seem different, and seem to change, but regardless there is one formula and one Being underlying everything. Zeno agreed with Parmenides. Zeno is famous for four main paradoxes, which he says describe the impossibility of motion. These four paradoxes fit the quadrant model pattern. They are
*Square one: the dichotomy paradox
*Square two: Achilles and the tortoise paradox.
*Square three: The Arrow paradox.
*Square four: The Stade paradox. The fourth paradox is different from the previous three. This is the nature of the quadrant model pattern.

Plato was concerned with the creation of an ideal society. He said that governments undergo transformations. His description of the process of government change fits the quadrant model pattern. He calls this the five regimes.
*Square one: Timocracy--related to the Idealist. Plato says that timocracies are nice. The people in timocracies act as though they do not care about gold. Idealists often act as though they are spiritual and do not want to take part in the physical world. But idealists also want to belong, are conservative, and like the status quo. Plato says that people in timocracies secretly worship gold, but will not admit it. Socrates describes that that timocrats work on cultivating their virtues and intellects. This is the nature of the first square. But at the same time they are not content. Therefore timocracies become oligarchies. Asians are related to timocracies, sometimes acting as though they are not greedy, and are spiritual. Asians are the first square ethnicity.
*Square two: Oligarchy--distinguishes between the rich and the poor, making the rich their administrators. Keirsey's Guardians are related to oligarchies. Guardians are all about order and homeostasis. Guardians respect authority, hierarchy, and order. Guardians tend to be wealthy because they plan and are organized. Socrates thought that oligarch rulers make small armies so the poor will not rebel against them. Therefore oligarchies deteriorate because outside forces are too strong. Oligarchies degenerate into democracies.
*Square three: Democracy. The third square is about fun and doing. The third square is spontaneity. The third square is related to Keirsey's Artisan. Socrates describes that the supreme good in a democracy is labelled as freedom, but the poor get poorer and the rich get richer; everything becomes a popularity contest, and things are not decided by actual competence. Socrates says in a

democracy diversity is supreme, and people are allowed to do whatever they want, which leads to the downfall of democracy, as people begin to consume things that they do not need, becoming hedonistic, pursuing their desires and lusts, and nothing else. Inevitably disillusionment with democracy follows, creating an opening for a tyrant to emerge. Socrates says that the tyrant will be a smart man who is a philosopher. This leads to the fourth regime, the tyranny.
*Square four: Tyranny. The fourth is different from the previous three. The fourth is related to the Rational personality type. Rationals are philosophers, This leaves a power vacuum where a philosophical, intelligent tyrant seizes control. The tyrant must kill his enemies, which makes many enemies. Then the tyrant has to protect himself and his family with a lot of body guards. There are many people who want to kill him, and in order to not be killed the tyrant has to continue to kill his enemies. But in so doing he makes more enemies. The tyrant has such complete power that he begins to act lawlessly, becoming extremely blood thirsty. Socrates says that out of the tyranny emerges an aristocracy. The forth square is related to the philosopher and can be seen as bad.
*Square five: Aristocracy. Plato describes this as the ideal form of government, transcending all others. The fifth is related to God; the Kingdom of God. He makes a proposition of how a society can run in a three caste system, but realizes that this too will deteriorate. Finally he gives a metaphor of a perfect society, picturing each of the three castes as a type of animal. He says one is a human, one a lion, and one a bird. He said to put these three animals within a human, which represents the form of an ideal society. What Socrates described was the quadrant model pattern. Socrates put three elements within a fourth element; that is the nature of the quadrant model. In it there are three squares that are consumed by a fourth square. It is also interesting that philosophers note that this image is like a fractal image. It can be proposed that the human that these three elements are in is in another human with another lion and eagle. Then that human is another human with another lion and eagle next to it--so on and so forth, forever. Fractal mathematics is integral, physicists think, in the construction of reality. It is interesting that Plato may have intentionally drawn a fractal.

Plato proposed the existence of four virtues that people possess. These virtues fit the quadrant model pattern. They are
*Square one: Wisdom. The first square is always related with the mind. This is related with Keirsey's Idealist temperament.
*Square two: Temperance--related with the Guardian. Temperance is the ability to control oneself.
*Square three: Courage. This is related to the Artisan temperament. Courage is related with doing. The third square is doing. Courageous people are willing to put themselves on the line, doing things that are dangerous, and often considered bad or destrictive.
*Square four: Justice--the consequence of the harmony of the other three

virtues. In other words, Justice involves the other three virtues. The fourth is always different, yet encompasses the previous three. Where there is synergy between wisdom, temperance, and courage, there is justice. Justice is related to the Rational temperament.

Aristotle, a student of Plato, is the third square philosopher of Greek Philosophy. Plato searched for an ideal form of government, and evoked a world of Forms for being the organizing principle behind reality. Aristotle used both his intuition as well as his experience to describe reality. He was very prolific in his ideas, even studying olympic athletes who won olympic events. Among other things, he is known for his four causes, which fit the quadrant model pattern. These four causes are causes of movement.
*Square one: Material cause--determined by the material of which it is composed. The material cause of a basketball might be rubber.
*Square two: Formal cause--related to the arrangement of a thing. Aristotle uses the example of octaves, and the 2:1 ratio of the string being the cause of the octave. The formal cause of a basketball might be its density and the way its texture is arranged. The second square is order and structure--the nature of the second square.

*Square three: Moving cause/efficient cause. The third square is the doing square; it is always related to movement. The moving cause according to Aristotle is something apart form the object being moved; it is interacting with the object, causing it to move. The efficient cause of a basketball may be a basketball player.
*Square four: Final cause--the purpose for which something is being moved. The purpose of moving a basketball is to have it go through a net. The purpose of a bullet might be to kill a living organism. These four causes inspired Tinbergen's four questions. Aristotle argued that there must be a primary mover that caused everything to move. Aristotle says that for every effect there was a cause, and there must have been a first cause. This, some scientists say is corroborated by the big bang theory. Physicists who study the multiverse and M theory suggest that the first cause might have been two primordial membranes colliding, the product of which was the big bang. Others say that this Universe was created from another Universe in the multiverse, which leaves a question regarding what created the multiverse that created this universe. Still Aristotle says there needs to have been a primary mover. Many have taken this to mean that Aristotle believed in a God that started everything.

The “9/11” disaster reveals the quadrant model as follows:
*Square one: World trade center tower one
*Square two: World trade center tower two. These two are the duality. To many throughout the world the world trade center represents greed and empire.
*Square three: Pentagon. The third is the most solid; it is a war building and a building for the United States military.
*Square four: The fourth plane landed on the ground and disappeared. The fourth always seems not to belong with the other three. The fourth always seems different and almost as if not to exist.

The Quadrant Model of Reality is a revolutionary book. It represents a paradigm shift in the way that people view reality. No longer can it be argued that reality is random. The Quadrant Model of Reality is a sort of theory of intelligent design. It states that nature is manifested around a principal pattern. This pattern is elucidated in the theory the quadrant model. Things in reality are often structured in layers or compartments or sections. There is often continuums in reality, but these continuums are also broken down into discrete sections. The quadrant model of reality argues that the ordering and structure of reality is not random but is based on a specific pattern. For instance the layers of the atmosphere are four and each level has certain qualities and characteristics. The layers of soil are also four and each layer embodies certain qualities and characteristics. The stomach is divided into compartments and each compartment has distinct characteristics. The particles of the Universe are ordered in a model called the standard model of particle physics. The quadrant model argues that the structure of the standard model, the atmosphere, the soil, the planets, the human eye, the forces of nature, the bible, the Vedas, and everything that is important is not accidental and random like a lot of scientists say, but is purposeful and based on a pattern, which is called the quadrant model pattern. Each square in the quadrant model embodies certain qualities and characteristics. There are 16 squares in all, and four quadrants in all. The book The Quadrant Model of Reality proposes that reality is organized along the basis of the quadrant model pattern. This book is a groundbreaking, earth shattering work that signals a brand new perspective on existence that completely defies and overpowers and subsumes any theory created before it, and the quadrant model theory is to this point the most accurate and successful explanation of the nature of existence. It seems almost impossible to believe that one simple model can explain all of existence exhaustively. Many have stated that they have come up with such theories, successful theories of everything, but they have not. There is a movie called the theory of everything describing Stephen Hawking's life, but his theory of everything is not really a theory of everything. His theory of everything is a theory of physics only. The quadrant theory of everything includes his theory within it as well as, well, everything else that is not in the domain of physics. The quadrant model of reality is the closest thing to a theory of everything ever devised. It seems unbelievable that there really can be one simple theory; one principle; one model that can explain existence perfectly and succinctly. But there is, and that theory is the quadrant model of reality. Hopefully you enjoy. Because this book signals a global consciousness shift, a sort of enlightenment, and one that is much needed in a world that many find to be confusing and overwhelming. And people are searching for answers and a way to make sense of a world that they are growing to believe is senseless. The quadrant model proposes that things aren't so complicated and complex as many would like you to believe, but that everything is centered around one organizing principle, and the cosmos which may seem to be random and chaotic, is really ordered beyond you can imagine. No this theory is not wishful thinking, or an attempt to simplify the cosmos in a way that is not intellectually honest. There is no attempt in this book to try to cram things conveniently into a theory where they don't belong. The theory doesn't involve searching for patterns and trying to force patterns where there is none. The quadrant model is not another example of Jim Carey's movie 23 where Mr.Carey is trying to prove his theory that the number 23 is everywhere and as a result tries to find the number 23 everywhere and believes he does. The quadrant model of reality is a descriptive theory of nature that proves the famous Ockam's razor- the most simple, elegant, and beautiful explanation is the one that is most likely to be correct. The quadrant theory is a metaphysical theory on the nature of being, and it answers the most important questions ever asked- What is being and why is being the way it is? Why do we exist and why do things exist the way they are? And the quadrant theory answers these questions in an amazingly simple way, and the simplicity of the model in no way detracts from its extraordinary accuracy and descriptive power. The quadrant model pattern is in fact the formula that underlies all of reality, and is the formula out of which reality reveals itself. The quadrant theory is the ultimate revelation, the final revelation of existence. This theory is the apocalypse- nothing has ever surpassed this and nothing ever will.

The above four scenarios fit the quadrant model pattern, providing discernible evidence of the underlying Form of Existence.
In the legend of Jacob the twelve sons are the patriarchs of the twelve tribes of Israel. The order of the sons fits the quadrant model pattern. The Form of Existence is revealed consistently in the quadrant model patterns found in the stories of religions. In Quadrant 1 are Leah's children; Leah is not loved by Jacob. He loves Rachel, but produces the first four sons with Leah, all of whom are named in relationship to a perception (the first quadrant includes sensation and perception. This is no coincidence. The ordering of the children is meant to reveal the quadrant model pattern.
*Square one: Reuben—meaning, "the Lord has seen my misery". The first square is the light, which is related to sight. Seeing is associated with the mind and is more spiritual. The first square is the science square.
*Square two: Simeon—meaning, "the Lord has heard that I am not loved". The second square is the word, and hearing. Hearing is more relational, and is the religion square.
*Square three: Levi—meaning, attached. Leah says "Now at last my husband will become attached to me, because I have borne him three sons". This is related to the sense of touch. The third square, the art square, is the body square--the flesh.
*Square four: Judah—meaning, praise. After having Judah, Leah says, "This time I will praise the Lord.” The fourth sense is the sense of taste, which is related to the mouth. The fourth square, the philosophy square, is the true word. This is the philosophy square.
Following the account of Leah's four children there is a demarcation in the action, signaling the beginning of a new quadrant. The first four sons are all related to senses--the first quadrant is sensation and perception. Quadrant 2, connected to relationships, is belief, faith, behavior, and belonging. The first quadrant has a connotation of not belonging--Leah wants to belong, but is not loved by Jacob. Rachael, who does belong, but has no children, offers her servant, Bilhah, to Jacob to bear children in her behalf. The children of this relationship are:
*Square one: Dan--meaning vindication. Rachel says, "God has vindicated me; he has listened to my plea and given me a son". This has a connection with belief, which is the first square of Quadrant 2. To ask for something, believing that it can happen, it happens
*Square two: Naphtali means struggle. “I have had a great struggle with my sister, and I have won”--a statement about their relationship. This square is about relationships.The second square of the Quadrant 2 is faith, which is a

struggle. Faith is the most relational.
*Square three: Gad means good fortune. Leah says, "what good fortune I have" when he is born. Fortune is connected with doing. Also Quadrant 2 is positive, related to harmony, and always conservative. Fortune is good and leads to a conservative lifestyle.
*Square four: Asher means happy. Leah says "How happy I am! The women will call me happy.” Happiness is associated with belonging, which is the eighth square--the fourth square of Quadrant 2. Leah is relating herself with other women, making herself appear to belong. Evolutionary psychologists point out that women can see children as a symbol of success--having more children leads to belonging. This square is associated with belonging.
A pattern emerges in the order of the names of the sons of Jacob. Quadrant 2 is related to servitude. Belief, faith, behavior, and belonging have a quality of servitude. To have faith and behave is to follow orders. To belong is to fit into a group. Belonging connotes property--belonging to another is like being their property. There is a distinction between the first two and the second two squares; the first two are more conservative, the second two more destructive or “bad”.
Quadrant 3 follows, which includes the next four children, the first two by Leah, and the second two by Rachael.
*Square one: Issachar--means reward. Leah says "God has rewarded me for giving my servant to my husband". Reward is associated with action. Quadrant 3 is the action quadrant--thinking, emotion, doing, and dreaming. Thoughts manifest reality, one form of which is a reward.
*Square two: Zebulun--means presented with a precious gift. Leah says, "God has presented me with a precious gift. This time my husband will treat me with honor, because I have borne him six sons". Quadrant 3 is about honor; Artisans are the third quadrant. They want honor and respect. Here God presents Leah with something precious, which gives her honor.
*Square three: Joseph. Rachel is the mother of this son. Again the first two squares are conservative, while the second two are different. Leah has the first two sons of the second quadrant. Rachel finally has a son here and says "God has taken away my disgrace.” “May the Lord add to me another son.” The third square is action. Taking away disgrace is an action. Quadrant 3 is also about respect. Disgrace is disrespect; Rachel now has respect.
*Square four: Benjamin. After giving birth to Benjamin Rachel dies. The fourth square of the Quadrant 3 is dreaming. Dreaming leads to Quadrant 4, which is death.

In another legendary narrative Samuel has an experience that fits the quadrant model pattern.
*Square one: Samuel is asleep and is called by God. He runs to Eli and says "Here I am; You called me". But Eli says, "I did not call you go back and lie down".
*Square two: Samuel hears the voice of God and again runs to Eli saying, "Here I am; You called me". Eli says "I did not call. Go back and lie down". These two first squares are very similar, forming the duality.
*Square three: God calls Samuel a third time. Again Samuel goes to Eli with the same response. But Samuel says, "Go and lie down, and if he calls you, say, ‘Speak, Lord, for your servant is listening.’” In other words, “Take action—do something”. The third square is doing.

*Square four: This time Samuel hears the Lord say, "Samuel; Samuel". This time, the Lord is reported to have come and stood there, calling as at the other times. Then Samuel said, “Speak, for your servant is listening.”' The fourth transcends the previous three; it is responsive directly to God. The fourth is always different from the previous three.

In the legendary narrative of Abraham God makes a covenant with Abraham, telling him to complete it by offering four sacrifices—with a possible fifth.
*Square one: a heifer. This is a young female cow that has net yet had a calf. The first two squares are more feminine and conservative. The second two squares are more destructive. They are more masculine.
*Square two: a goat, a less solid, less physically imposing, more feminine-like animal, connoting order and homeostasis.
*Square three: a ram, a more solid, physically imposing, masculine-like animal. Rams compete violently and destructively for dominance, using their solid horns. They are doers, which is the nature of the third square.
*Square four: a dove. The fourth is always different from the previous three, seeming not to belong. The fourth offering is a bird; the previous three are mammals. Also the dove is not cut in half whereas the previous three are.
*Square five: a pigeon. The fourth always indicates and points to the nature of the fifth. The fourth is the dove--a bird--the pigeon is also a bird. It also is not cut in half.

Physical existence reveals itself as an illusion, which reveals an underlying pattern or form. One essential way in which it is revealed is through religious myths and legends. For example, in the mythical story of the Flood Noah releases birds on four occasions.
*Square one: Noah sends a raven that flies back and fourth until the water on the Earth dries. The first square is the air. The raven stays in the air until the water dries. So this raven is associated with the air. The first element of Aristotle is the air.
*Square two: Noah sends a dove to see if the water has receded, but the dove finds no place on which to perch, and must return to Noah--water continues to cover the face of the Earth. The second square is associated with water, as the first square is associated with air. Aristotle's second element is water.
*Square three: Noah sends another dove, which returns with a freshly plucked

olive branch in its beak, signifying that the land has reappeared--there is Earth. The third square is always associated with land. The third square is always associated with Earth, with physical and solid attributes. Aristotle’s third element is Earth, the doing quadrant. The dove is doing an action—third square.
*Square four: The fourth dove sent does not return. The fourth square is different from the previous three, having a somewhat transcendent quality, like it does not belong.

If you read my book the quadrant model of reality you will understand all things in existence. I brought the Truth to the world I brought the light to the world I brought the ultimate revelation.
For instance if you read my book you will understand why there are the four world religions, and why the nature of these religions are the way they are.
Here is an excerpt from my book the Quadrant Model of Reality.

There are four world religions. Some sociologists argue that there is a fifth; Judaism is sometimes considered the fifth, but is most often not included among the world religions because it is an ethnic phenomenon that does not try to convert people. The recognized world religions are:
*Square one: Buddhism--associated with the first quadrant, it is stereotypically about sensation, perception, response, and awareness. It is focused on finding the real self, which is the nature of the first quadrant, and is known for meditation and other activities related to perception, and awareness. Idealists, the first square personality type are often attracted to Buddhism. The Buddha was an Indian. He taught people to get married, have children, not commit adultery, not murder, and not steal. His teachings are very similar to the teachings of the Torah. Buddhism is the first square, and Christianity, which follows the Torah is the second square. So they are similar and there is overlap. Buddhism and Christianity are the duality. Some Buddhists deify the Buddha often praying to the Buddha, like Christians deify Christ. There are stories of the Buddha walking on water and dyeing and resurrecting, and there is many similarities between Buddhism and Christianity, although Buddhism, as the first square, is more mental. Buddhists are taught that life is suffering, so they can be sad, an emotion of the first square. The buddha taught that people should seek nirvana, which is separation from the world, and from a destiny of rebirth. Buddhism is associated with asians which is the first square race. Buddhism is associated with non violence and peace which are idealist characteristics.
*Square two: Christianity--associated with the second quadrant, is about belief, faith, behavior, and belonging. Christians are taught that "believing in Jesus" brings salvation. Messianic Jews teach that Jesus was an orthodox Jew who taught others to follow the Torah precisely. So Christianity can be connected to behaving, although Christians tend to emphasize belief. Messianic Jews teach that Paul was also an orthodox Jew who sought to bring the Torah back to the lost tribes of Israel, whom he called gentiles, because they had broken out of covenant--gentile means out of covenant. According to messianic Jews, Black Hebrew Israelites, and even Seventh Day Adventists, Jesus and his disciples taught that belief in Jesus entailed following the commandments of God; the second square focuses on order and homeostasis. Christianity is second square oriented, and associated with the Guardian personality type—wanting to belong. Christianity is characterized a lot by trying to convert people and save people, which is associated with wanting to belong, and it is not known for being related to deep thinking, but more belief, which is characteristic of the second square. Christianity is associated with Europeans and is the second square race.
*Square three: Islam--a third quadrant religion. Like Christianity, Islam considers itself an Abrahamic religion--descended from Abraham. Arabs consider themselves descendants of Ishmael, a son of Abraham. The Israelites descend from Abraham's other son, Issac. Some rabbis think that Europeans are often descendants of Issac's son Esau; Israel descends from Issac's other son Jacob. The third quadrant is thinking, emotion, doing, and dreaming. Thinking challenges beliefs; Islam challenges the beliefs of Christianity, teaching that Jesus is not God, but is a messenger of God. Thinking is considered to be destructive and bad for challenging and breaking down beliefs, and breaking Christians out of the comfort of their beliefs. Islam means submission to God. Islam is often associated with Black people and arabs, which is the third square race. Also Islam is associated around the world as being violent, and the nature of the third square is it is more “destructive” or “bad”. The third square is conservative. I discussed that in the Nolan Chart, the third square is the conservative. Conservatives believe in economic freedom but do not believe in personal freedom. Muslims you will see with large cats/lions and fancy cars and following economic freedom. There is the saying "Arab money". They will flaunt their wealth in ostentatious displays, which is something you will not so much find Buddhists doing. But you will also find them against personal freedom like homosexuality and promiscuous women. Christians on the other hand are second square oriented which is fascist. The second square of the Nolan chart is no personal freedom and no economic freedom. Christians do not believe in economic freedom or personal freedom. Muslims will have teachings where they teach that Muhammad BPBUH taught rich people to dress according to their wealth and allowed for economic freedom, whereas there is more of a proclivity among Christians to look down upon wealth and economic freedom. As fascists, Christians also look down upon personal freedom, so they too are against homosexuality and promiscuity of women. Buddhists are the first square. The first square of the Nolan chart is communism which is for personal freedom and against economic freedom. So Buddhists are more for personal freedom, but against economic freedom. As a result, Buddhism is the opposite of Islam. Asian Buddhists and Black Muslims are opposites. So Buddhists are more against extravagant displays of wealth. Buddhists try to see the world as illusory, and they perceive attachments to material things as entrenching one in the world of attachment. Buddhists are less judgmental than Muslims and less desiring to force people to obey commandments, and thus more for personal freedom, whereas Muslims are very much against personal freedom. Because Buddhists try not to stay attached to things, they are not so absorbed with forcing women to not be promiscuous by covering their faces with masks and so on. Muslims are conservative, and they want to try to take away personal freedom and bash women who are "whores" and "sluts" by not covering their whole bodies and leaving the house or driving, and despise homosexuals, and force conformity and dominance of men over women. Buddhists will not go around in cars hunting homosexuals to kill like Muslims might. Muslims will go on patrols searching for women who are not completely covered and without a male chaperone and they will rape them and harass them and call them out, as if the Muslim men cannot control their sexual urges and their desire to have sex with any woman in sight if she is not protected by a man and completely covered. Such a tendency is a conservative tendency, and is characterized by the third square. A Buddhist, who does not want to be attached to the material world and imprisoned by material stimuli would not involve himself with such preoccupations. Whether Muhammad BPBUH taught to hunt homosexuals or not or patrol for women who do not have their bodies fully covered is not important. I am looking at what Muslims do and what they do not do and what Buddhists do and do not do. And you will find Muslims doing what is characteristic of conservatives on the Nolan Chart and Buddhists doing what is characteristics of communists on the Nolan Chart. The first square Buddhism communists is opposite of the third square Islam conservatives. Whereas Buddhists will be more inclined to have authentic true respect for women and homosexuals and be less judgmental, Muslims will have more of an inclination to be patronizing towards women and objectify them and be more hateful of gays, and absorb themselves in enforcing stratification and lack of personal freedom. Asians tend to be Buddhists, and Black people tend to be Muslim. Asian is the first square race and Black is the third square race. Muslims are the artisan personality type.
*Square four: Hinduism--a “polytheistic” religion, Hindus tend to believe in more than one God, and worship different Hindu Gods. The fourth quadrant

encompasses the previous three, while pointing beyond them. The fourth quadrant is contemplation, passion, flowing, and knowing. Hinduism is definitely the most contemplative of the world religions. Also Hinduism encompasses the other world religions, teaching that the messengers of other religions, like Jesus, and Muhammad, and the Buddha, are avatars of the Hindu God Vishnu or other Hindu Gods. So Hinduism is more pluralistic, containing the previous three. Although, there is wide variety in the fourth square, as it encompasses everything in the previous three. The fourth square always encompasses the previous three. Some Hindus believe that the ultimate awareness is that humans are Gods. Hinduism is associated with
karma sutra, which is a type of meditation based on sexual positions. The fourth quadrant is knowledge; knowledge is associated with sex. Again, the Nolan Chart characterizes the fourth type, libertarians, as for personal freedom and for economic freedom. Practices like Karma Sutra demonstrate personal freedom. Islam and Christianity are against personal freedom, so you will not find practices like Karma Sutra which teach sexual freedom espoused by them as much. I discussed there are four emotions according to scientists. Hinduism is associated with fear. Contemplation is the fourth quadrant. Fear emerges from contemplation, where you step out of beliefs and thinking and let go of the ego and contemplate. It can be scary to contemplate, and Hindus are stereotypically more connected to contemplation than belief. Christianity is related to belief, and is the second square, and contemplation is related to Hinduism, the fourth square. There are four emotions I described. Fear is the fourth square emotion. Hinduism is associated with Brown people/Indians, which is the fourth square race. The fourth square is also known for being “bad”. Both Christians and Muslims tend to look down upon Hindus seeing them as polytheists, although there are Hindu sects that are considered monotheistic. Again, Buddhism is less judgmental, and will not condemn Hinduism for polytheism, as Buddhists themselves will worship multiple Gods or Buddhas. Again, Buddhists and Hindus are more for personal freedom and less concerned with commandments compared to Christianity and Islam. Christians and Muslims will condemn yoga, seeing it as too spiritual and connecting people to Hindu Gods. Christians and Muslims may even argue that it gives women too much freedom, allowing them to wear tight yoga shorts and show off their butts in downward facing dog and other "sexual" positions in front of men. Such a practice would be inconceivable for Muslims, and would be looked down upon by Christians as well. The Muslims would act as though they are highly agitated by the scantily clad women and they cannot contain their urges and they would maybe stare at the women's butts and then rape them and then say that the women deserved it. They would maybe argue that the women wanted it and call them sluts. The men would say that they were perturbed by their butts and could not control their desires and the women need to cover up and have a chaperone male to protect them. One cannot imagine Buddhists acting like this but it is very conceivable to picture Muslims doing this because Muslims do this. Again Muslims are conservative, whereas Buddhists are not as conservative. Even Buddhists look down upon Hindus seeing them as not accepting the teachings of the Buddha. The fourth square is bad to everybody, and does not seem to belong, so even Buddhists look down upon Hindus. The first square tends to not be as judgmental as the second square though, because the second square is very much tied to homeostasis and order, so Buddhists however will not judge Hindus as much as Christians will judge Hindus.. Hindus tend to say the Buddha was an incarnation of God, but that the Buddha did not contain full revelation and purposefully lead people astray. The Mormons considered Christians argue the bible is also polytheistic, so there is connection between Hinduism and Christianity, as there is connection between Hinduism and all of the religions. However, Hinduism and Christianity are opposites. Hinduism is the fourth square and Christianity is the second square. Hindus are for personal freedom and economic freedom, whereas Christians are against personal freedom and economic freedom, typically. Some Hindus reconcile all of the religions, saying that they believe in Allah as the only God, but they will say that Allah is Krishna or Shiva, or something like that. Some Hindus will believe that Krishna is the Father of Jesus, and that the Buddha was an incarnation of Krishna. Hindus have many creative ways of reconciling all of the religions. Hindus are for economic freedom and personal freedom. Hindus have arguments such as "if you are rich that is because you were born into that life and it is a blessing by the gods due to your merit and you deserved it due to past lives of reincarnation where you achieved your stature". Hindus will accept more economic stratification and showing off of wealth like Muslims, and unlike Christians and Buddhists who have teachings more geared toward economic equality for all (no economic freedom/fascism) and not displaying and boasting about riches and stratification. Buddhists and Christians are related to communism and fascism, whereas Muslims and Hindus are connected to conservatism and libertarianism. Hindus are the rational personality type.
The World Religions

Regardless, Religion is the second square field of inquiry. Science is the first and art is the third and philosophy is the fourth. I described how four fields of inquiry were elucidated. So religion is going to be conservative and about homeostasis regardless what religion is being described, because the nature of the second square is homeostasis and maintaining order. There are just degrees and differences within different religions that correspond to the quadrant pattern because the quadrant pattern is the foundation of existence.